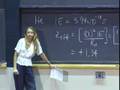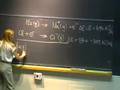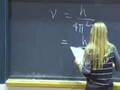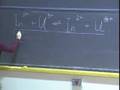Flash and JavaScript are required for this feature.
Download the video from iTunes U or the Internet Archive.
Topics covered: Matter as a Wave
Instructors/speakers: Prof. Sylvia Ceyer
Lecture 5: Matter as a Wave
Related Resources
The following content is provided by MIT OpenCourseWare under a Creative Commons license. Additional information about our license and MIT OpenCourseWare in general is available at ocw.mit.edu. All right. Last time, what we had done is that we had looked at the first evidence for the particle-like nature of radiation. And that evidence was a photoelectric effect. The evidence was that what you had to have was a photon or a particle of energy, a quantum of energy, a packet of energy, in order to get an electron out. And that energy had to be at least the energy of the work function of the metal. And so for every packet you put in there, you got one electron out. That is an example of the particle-like nature of radiation. But Einstein went on to show an even more convincing property of the particle likeness of radiation or a photon. And that is that what he did was showed that a photon has momentum. It has momentum, even though a photon does not have mass, although a photon does not have rest mass, for those of you in the know in this area. And having momentum is very much a particle-like property, right? Because you know how to write down momentum. Momentum is mass times velocity. You've got a mass in here. That is a particle-like property. And, yes, I am starting out with the lecture notes from number four, which I didn't finish last time. That is a particle-like property. But what Einstein showed was, from the relativistic equations of motion, what drops out from the relativistic equations of motion is the fact that a photon, at a frequency nu, has a momentum h nu over c. And because we know the relationship between nu and c, nu times lambda equals c, I can write the momentum of a photon as h over lambda. If you have some radiation, this is the photon momentum here. If you have some radiation, at a wavelength lambda, that radiation or those photons have this momentum p given by h over that wavelength. Now, that was a prediction from the relativistic equations of motion. And it took another eight, ten years or so before there actually was an experiment that demonstrated the momentum of a photon. And that experiment was called the Compton experiment. What went on in that experiment is that an X-ray beam came into some material or some molecule, some atoms, and they could actually see the transfer in the momentum from the photon to the atom. Kind of like in this website from the University of Colorado, here. This is just a cartoon of what is happening, but I got this photon done and I got this atom coming at me. And I cannot move this fast enough. I am going to get clobbered. You have a different computer than I have. Oh, I have to push down. Okay. Well, if I get aimed here, these photons are coming at this atom, coming at me. And, boy, if I do it fast enough, I can turn it around. Hey, I did it. But now, of course, if I go and lower the power. Come on. Come on. Aah! I got killed. [LAUGHTER] Christine, I don't like your computer. Oh, wait. I have got to get it. Get it. Get it. Get it. Please. Please. Please. Aah. All right. Well, you guys are going to be a lot better at this than I am. You can go and play with this. Christine is going to try now. Oh, look at that. She is going to get it. She is going to get it. She is going to get it. Yeah! [APPLAUSE] Three cheers for Christine. Oh, now it something else. This is going to keep going here. You need more power there. [LAUGHTER] Hey, not that guy. Fantastic. All right. And it is actually just this effect that was used by Steve Chu at Stanford and Bill Phillips at NIST and Cohen and Tanugi who provided some of the theory behind it. It is just that effect that they used to literally trap an atom in space. How do you do that? Well, you take an unsuspecting atom, and you bring in a high power laser beam coming out in this direction. And those photons transfer momentum, and they push that atom this way. But you are smarter than that, so you bring in a laser beam this way. And so you have momentum transfer this way and this way. You just trapped the atom in this dimension. And then you bring in a laser beam this way. Bring in a laser beam that way. You have trapped the atom now in this dimension. What is that? Your dog. That is not part of my lecture. [LAUGHTER] And then you bring in the laser beam this way. And so now you have constrained in the three dimensions. And so the atom is trapped in space by this photon pressure, by this momentum transfer. And this is called laser trapping. And these three gentlemen, whose names I gave you just a moment ago, are laser atom trapping and received a Nobel Prize in 1997 for this demonstration. But the other reason why this laser atom trapping was really so important is because it is actually the first step in another experiment. It is the first step in producing a Bose-Einstein condensate. What this laser trapping does is literally to slow the atom down or to cool the atom, because temperature and the velocity of the atom, the speed of the atom are related. The slower the speed, the lower the temperature. And to produce a Bose-Einstein condensate, you have to have bosons, which you lower in temperature. And ultimately, they condense. And the temperatures have to be on the order of micro-Kelvin. And so this is the first step in producing that Bose-Einstein condensate. This will bring you down to temperatures of, say, a Kelvin or so. And then there are lots of other techniques, a couple of other steps that bring you down to the microKelvin range. And then, finally, you can get the bosons to condense. And one of my colleagues in the Physics Department, Wolfgang Ketterle, also received the Nobel Prize for the formation of the Bose-Einstein condensate. I actually think he is teaching a recitation section in 8.01. Maybe some of you have him. You do? No, you don't have him. Okay. But you will be able to meet him and talk to him. Question? I'm sorry. Fantastic. Has he told you about this yet? Oh, he went to a conference. Okay. Well, you can imagine he is in demand. But you will see him, right? I hope. Very important effect here. We have radiation that is exhibiting both wave-like properties and particle-like properties. And, just in general, experiments where the radiation produces a change in the state of the matter such as the photoelectron effect. In photoelectron effect, the matter changes in the sense that an electron is pulled off of it. In those experiments, the radiation usually exhibits the particle-like behavior. In experiments where there is a change in the spatial distribution of the radiation, or where the radiation interaction results in a change in the spatial distribution of the radiation, that is when the radiation exhibits its wave-like behavior. And so it really is not appropriate to ask, is light or radiation a particle or a wave? The appropriate question to ask is, how does light behave? Does it behave like a particle or does it behave like a wave under particular experimental circumstances? And having both behaviors, this wave-particle duality of radiation is not a contradiction. It just is the fundamental nature of radiation, of light. You may think it is a contradiction because in your everyday experience, you either see a wave or you see a particle. But that is your everyday experience. And there are parts of nature that you cannot see every single day. And those deeper parts of nature have different rules. And you have to be accepting of those different rules. And so it is not a contradiction in terms. It just seems strange to you just because that isn't your everyday experience. It is the fundamental nature of radiation. Well, not only is that the fundamental nature of radiation, but the wave-particle duality of matter is also the fundamental nature of matter. And that is what we are going to talk about right now. We are going to move to matter, particles. The particle-like nature of matter is within your everyday experience, but it is the wave-like nature of matter that is not within your everyday experience. And so let's take a look at that. Suppose we did this experiment. That is, we had a nickel crystal. And these two atoms here are just two of the atoms on the surface of a nickel crystal. And we know the spacings between these two atoms in the crystal because we know the crystal structure of the nickel. That spacing is about 2x10^-10 meters. Naively, if you brought in a beam of electrons, particles, and we know they have mass. J.J. Thompson taught us they were particles, they had mass. But, naively, if you brought them in, you might expect these electrons to scatter isotropically. That is that they would scatter equally in all directions so that when they ultimately hit this screen here, this curved phosphor screen, and I changed the geometry here to a curved screen just so that it will be a little bit easier to analyze the geometry of this problem, which we are going to do in a moment, you might expect this screen to be lit up uniformly at all angles. Well, this is exactly the experiment that Davidson and Germer did in 1927, along with this gentleman, G. Thompson, George Thompson, son of J.J. Thompson. And J.J. Thompson actually did an experiment a little different than Davidson and Germer. I am going to show you the Davidson and Germer experiment here. But here is the same diagram that I had before, except that I made the nickel atoms a little bit smaller just so that this diagram would be a little bit easier to understand. I cleaned up the diagram, but kept the spacing between the two nickel atoms the same. And so Davidson, Germer and Thompson came in, scattered these electrons and looked how they scattered back. And, lo and behold, what they saw is that these electrons seemed to scatter back at a preferential angle. The angular distribution was not isotropic. Instead, it looked like the electrons scattered back at a pretty well-defined angle here, 50.7 degrees. And not only did they scatter back at that angle, they also scattered right back at themselves. Backscattered this way, so this scattering angle is zero degrees. And under some particular conditions, the electrons also scattered at a larger angle, here. But the bottom line is that the scattering pattern was not isotropic. There was a bright spot, lots of electrons scattered at this angle, a dark spot, no electrons scattered at this angle. A bright spot, dark spot, bright spot. This looks like interference phenomena, just like the two slit experiment. Bright spot, dark spot, bright spot, constructive, destructive, constructive interference, back and forth. That was their observation. How do we understand that? Well, it is looking like these electrons are behaving like waves. Suppose these electrons are coming in, so we have this constant stream of electrons impinging on our nickel crystal. Well, what is happening here is that when these electrons are reflecting back from the individual nickel atoms, these individual nickel atoms are kind of functioning like those little slits we saw in the two slit experiment. That is, they are scattering back as a wave. These electrons seem to be scattering as a wave, so isotropically in all directions. This semicircle around each one of the atoms, and I only show you two atoms here, each semicircle is the maximum of the wave front. It is the crest of the wave front. And then, as time goes by, of course, these waves propagate out. And then another wave front, another wave maximum appears and a distance between these two maxima is, of course, the wavelength. And as time goes on, they scatter further. And as time goes on, they still scatter. And they keep the propagating out until they reach the screen. And, lo and behold, on the screen you see a bright spot, dark spot, bright spot, dark spot. Interference pattern. Let's analyze this. Here is the diagram again. I just moved it over and cleaned it up again. I want you to look at this spot right in there. That is where we have the maximum of a wave scattered from atom one at the same point in space as the maximum of waves scattered from atom two. Constructive interference. Here is another point of constructive interference. Here is another point of constructive interference. Everywhere along this line, we have constructive interference, which results in a large intensity right at this scattering angle here, a bright spot. And we already know the condition for constructive interference. That is, in order to get this constructive interference, the difference in the distance traveled by the two waves that are interfering has to be an integral multiple of the wavelength lambda. Now, I use the term d instead of r, but it is the same thing for the condition for constructive interference, here. And if you went and analyzed what the difference in the distance was for this constructive interference along this line, you would find it was n equals 1. The difference in the distance traveled is one lambda. And, if you looked at the points of constructive interference along this line that led to this bright spot, the difference in the distance traveled will be two lambda. This is our second-order interference feature, our second-order diffraction spot. And, if you look at it along the center here, normal to the crystal, that would be the zero-order spot. d2 minus d1 is equal to zero lambda. All right. That is what looks like is happening. Now, what we are going to do is we are going to actually analyze this geometry a bit more. We didn't do so in the two slit experiment. We could have. We didn't. We are going to do it here. And what we are going to be after is if these electrons are acting like waves, then they have a wavelength. And we want to know what the wavelength is. Davidson and Germer wanted to know what the wavelength was. And we are going to use this scattering angle here, theta. This angle from the normal to where the electrons are scattering, that angle theta, we are going to use that information, theta equals 50.7 degrees, to back out the wavelength. And we know what the condition is for constructive interference. We just talked about it. Here it is, d2 minus d1. That is the wavelength we are after in this analysis. Now, what is d2 here? Well, the length of this line, d2, is the distance that the wave that scatters from electron two travels from electron two to the screen. d1 is the distance that the wave that scatters from atom one travels to the screen. That is what d2 and d1 are, here. Now, I am going to draw a perpendicular from atom one to this line d2. There is my right angle. Now, you can see, then, that this leg of the triangle is d2 minus d1, the difference in the distance traveled. That is this quantity here. That is going to be important. Now, you have got to convince yourself that this angle right here in the triangle is the same as this scattering angle. You can convince yourself of that pretty easily. Now we have a well-defined triangle. We know one length of it, we have measured the angle theta, and d2 minus d1 is something that we would like to know. Let's do a little geometry. The sine of theta, the sign of this angle is equal to the opposite length, which is d2 minus d1, divided by A, this distance between the two atoms in the nickel crystal. We have two equations. This is the equation that in theory should obtain for constructive interference. This is the equation that we set up given the particular physical geometry of our problem. These two (d2 minus d1)'s better be equal to each other. We have n lambda is A sine theta. Let's solve for lambda. We can do that. That is A sine theta over n. We already know what theta is, we know what A is, so what is n? Well, n is going to be one because this is the bright spot that is closest to the zero-order spot, which is always present at the normal angle there if you are coming in at normal orientation. So n is equal 1 so I can plug things in. And, when I do that, I find that the wavelength that I predict is 1.66x10^-10 meters. We've got this wavelength. Now, before I go on, I just want to point out that this geometry of the problem that I set up here is identical to the geometry in a technique known as X-ray diffraction. X-ray diffraction does not use electrons coming in, but uses X-rays, photons. And it is a technique that is going to be important to you if you do any kind of science involving materials or biological systems. And it is important because X-ray diffraction allows you to determine the structure, in particular of proteins, crystal proteins. You crystallize the protein, and you use this X-ray diffraction to get out the structure. And the reason why you want the structure of the proteins is because the structure gives you a hint as to what the function of the proteins are. And so in the use of X-ray diffraction, we don't go and calculate what lambda is. We already know what lambda is in X-ray diffraction. We know the wavelength of the incident photons, the X-rays. What we don't know in the technique of X-ray diffraction is the distance between the atoms in an unknown structure. And so in X-ray diffraction, we know the wavelength, and we can figure out what order it is and we measure the scattering angle. And we use that to determine the distance between the atoms. And, in that way, we back out the structure of the sample. Yes. We are just going to get there. We are going to do that. All right. Same geometry here. Now, this experiment of Davidson and Germer was really an important one because just three years before this, there was a prediction for what the wavelength of particles ought to be. And that prediction was made by this gentleman, Louis de Broglie. In his Ph.D. thesis, no less, what Mr. de Broglie did was that he looked at the relativistic equations of motion that Einstein wrote down and used to propose that a photon or radiation with a wavelength lambda had momentum p. Well, he took those same equations and said, well, these relativistic equations of motion apply to matter just as well as they apply to radiation. Therefore, if you have radiation with a wavelength lambda, you then have this momentum p for the radiation. This is what Einstein said. But he turned it around and said, if you have matter with a momentum p, well, that matter ought to have a wavelength lambda. He turned around Einstein's equations of motion and proposed that the wavelength of a particle be given by h over p where, of course, p here is the mass times the velocity. Fantastic. What a great Ph.D. thesis. I'm impressed. Now, let's see how well, as you can imagine, that predicts the wavelength that Davidson and Germer actually measured. We know we have 54 electrons coming into this nickel crystal. That is their kinetic energy, one-half m v squared. Kinetic energy can also be written in terms of the momentum. The momentum is p square over 2m. You can convince yourself of this. This is a good thing to know for doing these problems, that the kinetic energy is p squared over 2 times the mass of the electron. And so if you solve that, what you get for the momentum of the electrons is 4.0x10^-24 kilograms meters per second. And now I can take this momentum and plug it into the expression for de Broglie's wavelength, 6.6x10^-34 joule seconds, over the momentum, 4x10^-24. What do I get? 1.7x10^-10 meters. Absolutely the same as the experiment. De Broglie made a prediction. A few years after that, experiments demonstrated that de Broglie was absolutely correct in his prediction. What do we have here? We have matter, particles, exhibiting wave-like behavior. And, those particles can be measured to have a wavelength that actually agrees with a prediction, some theory, the de Broglie wavelength. And we also have another phenomena here, which I really enjoy, and that is Davidson and Germer and George Thompson. They demonstrated that electrons behave like waves. And what did J.J. Thompson do, father of George Thompson, well, he demonstrated that an electron was a particle. Here, we have both the father and the son talking about seemingly opposite behavior, but they are both right. How often does that happen? That, I think, is really amazing. But if matter is wave-like and if electrons can be represented by a wavelength, then what about your wavelengths and my wavelengths? We should have a wavelength. And we do. And just briefly, here, let's talk about what the wavelength is of a baseball pitched by Curt Shilling at 90 mph. What is that wavelength? Well, a baseball is five ounces. 90 mph. You can calculate the momentum. It is in your notes there. We will calculate, here, the wavelength. And what you are going to find is that it is 1.2x10^-34 meters. That is pretty small. What is the diameter of a nucleus? 10^-14, right. That is a good number to know. Here, we have a wavelength that is 10^-34 meters. Is that wavelength of a macroscopic size object going to have any consequence in our world? No, absolutely not. Why? Because in order to see any effects from this small wavelength, we are going to have to have slits or atoms that are going to be on the order of this close together. But there is no way that we are going to have two nickel atoms that are this close together or two slits in a two slit experiment this close together. And so for macroscopic objects, the wavelike properties have no consequence in this world. And that is simply because the mass is too large. It makes the wavelength too small to have any effects in our everyday lives. And it is actually -- Yes? Okay. Well, they are actually coming in as waves. They are behaving as waves. Remember my beach picture? I drew them coming in like a circle. But remember my picture of this barrier here on the beach, and I am laying here on the sand, and then the waves are coming in? Here is blue. These electrons, as they are coming in, really need to be thought of as these kind of plane waves. I drew them as kind of just particles. But you have to really think of them as plane waves and that they are reflecting off of these two atoms in the way that I just explained. Another question? The thing is that you cannot get that velocity slow enough to make the wavelength large enough to be of consequence. If you could then you would, right? You would see the wave-like behavior. But you cannot, practically speaking, get it to that extent. No. In this particular case, anything that is so massive, any smaller effects, like what you are talking about, exactly the point of observation is not going to have an effect on anything that is so massive. Pardon? Yes, your point of observation will have an effect on your interpretation of the experiment, if you are talking about something that has a much larger wavelength. Absolutely. In high energy physics experiments, for example. Good question. It is just this observation, here, of the wave-like behavior of electrons, of particles, that led to the interpretation, then, or led to the realization that maybe, you have to treat electrons as waves. Or maybe you have to treat the behavior of electrons as wave-like behavior. And that is what this gentleman Schrˆdinger did. He said, well, you know what? This gave him an idea. Maybe what is wrong is that an electron in an atom, I cannot treat that electron as a particle. Instead, what I have to do is treat is as a wave. I have to treat its wave-like properties. And it was that impetus that led him to write down a wave equation of motion. An equation of motion for waves. He realized, or he was guessing at the moment, well, maybe in the case when a microscopic particle has a wavelength that is on the order of the size of its environment, in that case, maybe the wavelength has an effect, makes a difference. For example, in the case of the electrons, we had a wavelength calculated, there, of 1.7x10^-10 meters. That is a wavelength that is on the order of the size of the environment of the electron, which is on the order of the size of an atom. Maybe in that case I have to pay attention to the wavelength. The reason you and I don't have to pay any attention to our wavelength is because our wavelength is 10^-30 meters or so. And that is much, much larger than the size of the environment. In this case, we don't have to pay any attention to our wavelength. But, for an electron in an atom, we have a problem, here. What did Schrˆdinger do? Schrˆdinger said, I have to write down a wave equation. An equation of motion for matter waves. And what is that equation of motion? Well, that equation of motion is H hat operating on Psi, and it gives us back an energy, E, times a Psi. What is this? Well, Psi, here, is a wave. I am somehow going to let my electron in an atom be represented by Psi. This is going to be a wave form. This is going to be a wave function. I am going to let my electron be represented by the wave function. Exactly how it is going to be represented by the wave function is something that I am not going to tell you quite yet. But it is going to represent the electron. This energy, here, that energy is going to turn out to be the binding energy of the electron in the atom. This thing, here, is called the Hamiltonian Operator. That Hamiltonian Operator is specific to a particular problem, and we will look at the Hamiltonian Operator for a hydrogen atom. But this operator is operating on Psi, and it gives you back a Psi, the same function, multiplied by a constant. That constant is the binding energy. Now, you think, well, let me just cancel this and this. But you cannot do that because this is an operator. This has some derivatives in it. Its operating on Psi gives you back the same function times the constant. Now, how did Schrˆdinger actually derive this equation, so to speak? Well, what he did was to just guess at a wave function. We are going to use a one-dimensional wave function. He is going to say, let me represent my electron by Psi of x equal to 2 a times cosine 2 pi x over lambda. That is going to be my wave function. Why not? And then he said, well, what I really need here is an equation of motion. I need to know how Psi changes with x. If you wanted an equation of motion, if you wanted to know how Psi changes with x, what would you do to Psi? Take the derivative. Let's take a derivative of Psi of x, with respect to x. That is going to be equal to minus 2 a times 2 pi over lambda times sine of 2pi x over lambda. That is an equation of motion. Now, this is actually kind of an equation of position. But it is telling us how Psi changes with x. But now, since that gave us some information about how Psi changes with x, how would we get the rate of change of Psi with x? Second derivative. Let's take the second derivative of that. Second derivative of Psi of x with respect to x, that is minus 2a times 4 pi squared over lambda squared times cosine 2pi x over lambda. That's pretty good, but now, what do you see in this equation? Psi. You see what I started with. It is recursive, right. You see the Psi of x here. Let me write that equation in terms of the original function. Second derivative of Psi of x with respect to x, minus 2pi over lambda squared times Psi of x. lambda)^2 Psi(x)** That is pretty good. You know where I am trying to go? I am trying to derive, so to speak, Schrˆdinger's equation. See, it is not very hard. Yes. Now, this equation right here, this equation is just a classical wave equation. The only thing I have done so far is take derivatives. I have done nothing else. I just took derivatives. It could represent any kind of wave. There is nothing quantum mechanical about it. But here comes the big leap that Schrˆdinger made. He substituted in here for lambda the momentum of the particle. In other words, if this is a wave equation and that wave has some wavelength, here, lambda. He said, well, if this is a wave equation for a matter wave, well, then I better get the momentum of that particle in this wave. And he knew how to do that because de Broglie told him how to do that. De Broglie said, lambda is equal to h over p. That is pretty good. What can I do here? Well, what I can do is I can rearrange this and write this in terms of the momentum of the particle. Second derivative of Psi of x with respect to x is going to be minus p squared over h bar squared times Psi of x. Now, let me explain what h bar here is h bar is a shorthand way of writing h over 2pi. If you don't know it already, you should know it. You are going to need it. That is h over 2pi, so this is h bar squared. Now, what do I have? Now I have a matter wave. I have an equation of motion. I have something that tells me how Psi moves with respect to X. The rate of change of Psi with X. And I had the momentum of the particle buried in here. From this form, I am going to get to that. And I guess I am going to get to that next Wednesday.
Free Downloads
Video
- iTunes U (MP4 - 111MB)
- Internet Archive (MP4 - 197MB)
Audio
- iTunes U (MP3 - 11MB)
Caption
- English-US (SRT)