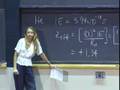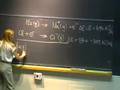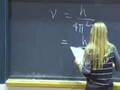Lecture 26: Molecular Orbital Theory

Related Resources

Molecular Orbital Theory (PDF)

• Download English-US transcript (PDF)

The following content is provided by MIT OpenCourseWare under a Creative Commons license. Additional information about our license and MIT OpenCourseWare in general is available at ocw.mit.edu. On Monday, we went through and looked at the functional forms for sp two hybrid orbitals, as found in the case of the BH three molecule. Now, you should recognize that there are other hybridization schemes that go along with different geometries. So, BH three was trigonal planar. And we are going to talk some more about BH three today. If you had been considering last time instead a tetrahedral carbon atom, then the hybridization scheme we would have needed to develop would have been the sp three hybridization scheme. That would be associated with a tetrahedral carbon atom. On the other hand, if we had a linearly coordinated carbon atom or some other main group atom, boron or beryllium, for example, then we would have had to develop the sp hybridization scheme. You should not miss the forest for the trees in putting into context from my previous lecture on the sp two specific case for that type of geometry. But now, rather than going through more examples of valance bond theory treatments of molecules, instead, it is time for me to introduce you to the tenants of the molecular orbital theory for treatment of molecular electronic structure. I mentioned valance bond theory as having been introduced by Linus Pauling. And then, I also named Robert S. Mulliken, MIT undergraduate, as the father of molecular orbital theory. And these are both two different perspectives on viewing electron structure of molecules that arises from the results of quantum mechanics. In the case of valance bond theory, we have a situation where you have localized electron pairs. In the case of molecular orbital theory, electron pairs are still going to be important, but they are going to be able to be delocalized over the entire molecule in some cases. So, this is very different. This is a major difference between MO theory and valance bond theory. In the case of the BH three molecule that we considered last time, you had four positively charged nuclei. And you arrange those four plus charges at the points in space that correspond to the equilibrium geometry of the molecule. And then you sprinkle in your six valance electrons, and you want to understand how those six electrons become organized in space in response to that electric field set up by those four positively charged nuclei. And we took the approach last time that we are going to localize electron pairs in between nuclei. And, because structurally the BH three molecule is symmetric and the three hydrogens are indistinguishable from one another, we decided that we were going to make hybrids. And so we talk about hybridization. The idea behind hybridization was to change the atomic orbitals of boron by mixing them, so that we would have one that would be able to point at each of the three hydrogens in space to form three nicely, perfectly directed electron sigma bonds between boron and hydrogen. That was the scheme that we adopted, this hybridization scheme, but in MO theory we are not going to do that. We are not going to interfere with the intrinsic atomic orbital structure of a boron atom in order to make bonds. And we are going to see that there are some predictions that come out of the MO treatment for the molecule that differ from those that came out of the valance bond treatment for the molecule. And so here, no hybrids. In terms of just the vernacular of chemical structure, you will hear sp three as being used interchangeably with the notion of a tetrahedron. But in valance bond theory, it refers to a particular hybridization scheme in which we actually mix s and p as a preparative to bond formation in a molecule. And we mix the atomic orbitals on that central atom in a hybridization scheme. In MO theory, we are not going to do that. It is very important that you keep these theories and the language associated with the theories separate in your minds so you can see the difference between these theories. And then one of the consequences of this valance bond theory and the hybridization scheme is that it is not so good for excited states. And what that means is that we were developing a scheme to describe the bonding in the molecule in its ground electronic state. Molecules can have excited states, just like atoms can have electronic states. And over here, in molecular orbital theory, we are going to find that we do a much better job with excited states. And that is important for understanding the range of properties associated with molecular systems. And you are going to see, indeed, that the energy level scheme for the six valance electrons in the BH three molecule is different, depending on whether you use valence bond theory or molecular orbital theory. Now, for molecular orbital theory, we are going to need, again, like we do for valance bonds, to have some kind of a procedure for forming molecular orbitals, conceptually. And the first step in such a procedure is that you are going to want to analyze the three-dimensional shape of the molecule. And we do this, of course, when we talk about the valance-shell electron-pair repulsion theory for predicting molecular structure. We are going to look at the structure. And we want to identify, usually by inspection, sets of symmetry-related -- -- atoms or orbitals. And here, I am talking about atomic orbitals. I will come back in a moment and talk about what I mean by symmetry-related. This is a concept that can be put on a very nice, firm, mathematical footing. And, in fact, if you find this type of analysis interesting and would like to see more of the math that can help you to organize the results of quantum mechanics in terms of symmetry, then you will want to put the 5.04 subject on your calendar for the future. That subject is devoted, in large part, to the applications of group theory to chemistry and chemical problems. And symmetry plays a big role in that. And then, two, we are going to form combinations. And let me just further quantify this by saying we are going to form linear combinations -- -- of symmetry-related orbitals. One of the big approximations that we usually kind of take for granted in the molecular orbital theory of electronic structure is the LCAO approximation. I am just going to mention that parenthetically, here. This is that we can form molecular orbitals that will be linear combinations of atomic orbitals. This LCAO approximation arises from the fact that we can solve the Schrˆdinger equation exactly for the hydrogen atom, but for big molecules and many electrons systems, we cannot. And what we like to do is to take the atomic orbital wave functions, that is, atomic orbital here in LCAO, and use those wave functions in our approximation of molecular orbitals. We are saying that we can combine these atomic orbitals on the atoms that are in a molecule to form the molecular orbitals that will be able to spread out and delocalize over the entire molecule. This is inherent in the development of the theory that I am working on here. And you should see that this will pop up in a number of cases, but it is an inherent approximation that we are accepting. And then, three, we are going to combine the linear combinations from part two -- -- with central atom atomic orbitals. And that is what we will do. And if we have done that properly, we will have arrived at molecular orbitals for the system in question. Now, I want to take a little bit of time to go through each one of these steps in order to define the problem that we have for this alternative way of viewing the electronic structure of the BH three molecule. Now, why am I choosing the BH three molecule for this? Well, I am choosing it because it is an easy problem and one that is illustrative of the steps that go into forming molecular orbitals for a system. In your textbook, you will see that before you get to something like BH three, first diatomic molecules are considered. Those, I will show you on Friday, are actually a little trickier to understand than is the case with BH three. That is because in molecular orbital theory we have the same number of MOs as AOs. If we start out with a certain number of atomic orbitals that come into play by virtue of the atoms that are in the molecule, then that number of orbitals will be the same as the number of molecular orbitals that we will get at the end of the problem. They won't all be filled. We will have some that are empty. But we are going to have the same number of molecular orbitals as atomic orbitals that go into the problem. And so what that means is that the complexity of the problem is related to the number of MOs and, hence, the number of AOs. The complexity of the problem scales with the number of atomic orbitals in the problem. We actually call these our basis functions. And one of the things that John Pople talks about, if you have gone and looked at his video that I pointed you to in the problem set, he talks about the wonderful fact that our computational power has gotten so great, and will grow so much greater in the future, that we are able to computationally handle problems of the calculations of the properties of enormous molecules that bring into a problem an enormous number, a vast number of atomic orbitals. And so, a great computational power is necessary to apply molecular orbital theory, or even the more recent density functional theory, to problems of electronic structure that we need to grapple with in order to predict properties of molecular systems. And in the BH three molecule, which is, as I said, a simple problem of electronic structure and a nice illustrative one for today's purposes, we have, this is a seven orbital problem. Why is this a seven orbital problem? It is a seven orbital problem because boron has four valance orbitals, an s, a px, a py, a pz. And we have three hydrogens, each with their 1s orbital. We have our valance orbitals on boron. We don't count the 1s orbital on boron because that is filled and is a core orbital, and it doesn't get involved in chemical bonding. The valance shell for boron is the n equals two shell. We have 2s, 2px, 2py and 2pz. And we have three hydrogens, each with their 1s orbital. If we were to tackle right now the problem of the molecular orbital energy level diagram for a diatomic molecule like O two, dioxygen, we would find immediately that this analysis of how many atomic orbitals we have available would give us the value eight. And so it is a more complicated problem. It has one more atomic orbital than this problem, even though this one has four atoms because three of these atoms happen to be hydrogens, which only bring in one orbital to the problem. That is why I am choosing this. And then, in addition, I am choosing this because the consequences of the symmetry of the BH three molecule are that the orbital interactions that we are going to identify occur in nice pair-wise sets. And that makes things especially easy to see in terms of how chemical bonds arise in the context of MO theory for a problem like this. And so, to now go ahead and carry out step one for this, we are going to draw the molecule and try to identify sets of symmetry-related atoms/orbitals. And, before you do that, I just would like to show you an example of a highly symmetric molecule, because this notion of symmetry is something that, at this point in time, I really only want you to gain an intuitive grasp of, I am not going to quantify it. But, if you look at a molecule like the one I have placed on the left and right-hand screens, you will see that it is a large, round molecule. Actually, round, spherical things are the most symmetric things that we can think of. Here, you can imagine that this is a big ball with atoms located at various points on the surface of the ball. This is just a ball and stick representation of the C60 molecule, also known as Buckminsterfullerene. It is a geodesic dome-type of molecule. This molecule has the chemical formula C60. There are no hydrogens in this molecule. All the atoms are displayed. And each carbon atom sits in a position where it is adjacent to one five-membered ring and two six-membered rings on the surface of this spherical molecule. And that is true of every carbon atom on the surface of this whole molecule. As you go on in chemistry, if you go into the analysis of molecules using nuclear magnetic resonance spectroscopy, you will find that it is really important to be able to identify the symmetry of a molecule. And you will realize that the symmetry of the molecule is manifest in the nuclear magnetic resonance spectrum of a molecule. If you take the carbon-13 nuclear magnetic resonance spectrum of this molecule, a sample composed of this molecule, you will see that there is only a single C13 signal in the spectrum. And that is because all 60 carbon atoms are in an identical environment in this molecule. Every one of them feels exactly like everyone else. They are all equidistant from the center of this molecule. And they are all equidistant from their set of neighbors. And so, in that way of looking at a molecule, you would see that they are all equivalent. You may have encountered this molecule before, but this molecule's discovery -- -- was actually predicted initially from analysis of mass spectrometry data by Professor Smalley, a Nobel Prize winner whose efforts in this area have spawned off chemistries involving not only these nano-sized balls of matter, but also nanotubes made of carbon, and this whole area that we think of nano technology. Really a lot of it is dominated by the chemistry of new forms of carbon that arose with the discovery of Buckminsterfullerene. And that is just one example of a new allotrope of carbon that was discovered in recent years. This gives you an idea for symmetry. I could show you pictures, actually, of enormous biomolecules. There are large viruses that are composed of biopolymers, macromolecules that pack in a way that is symmetrical, so that you can see these things. If you view them in the right kind of representation, you will be able to see the symmetry in them. And one definition of symmetry that I would like you to take away from this picture is just that each of the atoms that are symmetry-related is indistinguishable. If you turn the molecule around and look at the atoms, those that are symmetry-related are indistinguishable from one another. And so, when we have a trigonal planer BH three molecule, is the boron symmetry related to any of the other atoms? No, it is not. What about this hydrogen? I labeled them last time A, B, and C. This hydrogen labeled C, is it symmetry-related to other hydrogens? Yes. And that is because of the symmetry of this molecule with these 120 degree bond angles on the planarity of the molecule. So, you have a set of three hydrogens. And their 1s orbitals are in space indistinguishable from one another. They are related by symmetry. And so, in this problem here, we have four orbitals here on the boron that are not symmetry-related. And then also incidentally let me point out that the boron atom is located at the center of gravity of this system. If atoms are going to be symmetry-related, they must not be located at the center of gravity of the system. Let's go over here and expand on these ideas. The method that I am going to develop here for forming the linear combinations has to do with thinking ahead, in this problem, to the fact that we are going to want to make linear combinations that have the correct symmetry to bond to atomic orbitals on the central boron atom. Let me draw the ones that are going to be relevant to this part of the problem. This one over here would be the boron pz orbital, so it has one positive lobe coming out of the board, negative lobe going back. Over here we would have the boron py orbital using the coordinate system that I had chosen last time, which is x up and y to the left. And then here we have the boron's px orbital. And then over here we have the boron's 2s orbital. And so, our challenge now will be to construct linear combinations, we are at part two, of the set of three hydrogen 1s orbitals that can match in symmetry the boron central atom's atomic orbitals. Last time, remember, for hybridization, we were making these orbitals mix with each other in order to point at the hydrogens. Now what we are doing is kind of an inverse concept. We are going to mix the hydrogen orbitals so that they have the right symmetry to interact with the central atom atomic orbitals. There is a nice parallelism here. Here is going to be our LCs. They key feature of the boron's 2s orbital is that it does not have any nodes. Remember nodes are surfaces. When you pass from one side of a node to the other, you get a change in sign of the wave function. And we indicate that change in sign by differential shading. The 2s orbital has no nodes whatsoever. And a way that we can construct a linear combination that has the same spatial, nodal properties as that boron 2s atomic orbital is as follows. We can involve contributions from each of the three hydrogen 1s orbitals. Remember this one is A, this one is B, and this one is C. This is going to be a linear combination of the three hydrogen 1s orbitals. I will write this one as follows. This one will be written as A plus B plus C. That indicates the 1s orbital on A, plus the 1s orbital on B, plus the 1s orbital on C. And, as we talked about last time, wave functions that we write should be normalized. And they should satisfy the unit orbital contribution rule. For normalization, here, I give a factor of one over root three for this linear combination formed as a symmetry match with the boron 2s orbital. You can see, I hope, what we are doing. We are projecting the nodal properties of the central atom atomic orbitals onto the linear combinations that we are forming. And we are going to form a complete set of three linear combinations in this way. Let's make one that has symmetry properties that remind us of this px orbital. On HA we are going to have a positive contribution to match the positive contribution of this lobe of the px orbital that points along the plus x axis. And then down here we are going to have contributions from hydrogens B and C. And they are going to be smaller, and they are going to be opposite in-phase. They are going to be opposite in-phase because we are building a linear combination that has a node approximately at the center of the system here, so as you go from positive x into the negative x region of space, the wave function changes sign to match the change in sign associated with the px orbital. We are projecting the nodal properties of px onto the linear combination of hydrogen orbitals that we are forming here. And this one, written in normalized fashion, will be root two over three, A minus one-half B minus one-half C. And, although what we are working with here are linear combinations of these symmetry-related hydrogen 1s wave functions, you are going to find that these coefficients on the atomic orbitals, that contribute eventually to the molecular orbitals, are going to come out as normalized and as unit orbital contributions. So that if we started out this problem with a single 1s orbital on HA, that will be entirely accounted for among these linear combinations and the molecular orbitals that we are going to make with them. Now, let's generate a linear combination having the nodal properties of py. And, in order to do that, we need to have a negative coefficient out here in the minus y direction. We are going to put in a contribution from HC as negative, like that, to match that. And then over here, we are going to make a contribution for HB that is positive. And then, noting that there is a nodal plane along the y,z-plane, which comes out of the board like this, so that we are always negative along minus y and we are positive along plus y. We match that here. And the coincidence of that nodal plane with the location of HA dictates no contribution from HA to this orbital, for reasons that actually we looked at last time, -- -- namely, that we cannot bring a hydrogen 1s orbital in here and also change sign on going half-way through that hydrogen 1s orbital, because s orbitals have to have the same sign everywhere. It does not contribute to this linear combination. And our normalized form for this will be one over root two B minus C. And then, if I were to ask the question, can I make a linear combination of the three hydrogen 1s orbitals that has the same nodal properties as pz, the answer would be no because they all lie in the x,y-plane and they are just s orbitals and cannot change sign as you go through the x,y-plane from plus z to minus z. And so, we are done here. And what you are going to find is that we have created these three linear combination according to step two. And taking into account both the symmetry properties of the molecule to identify a set of three symmetry-related atoms and orbitals. And then, taking into account an analysis of the nodal properties of the central atom atomic orbitals, so that we could project those out to help us find appropriate linear combinations for mixing with the central atom orbitals. And, when we do that mixing, we are going to find out that there are three ways that we can do it. We are about to move onto step three of this problem. We are going to need to combine these linear combinations with the central atom atomic orbitals according to the rules of MO theory to generate, first, bonding molecular orbitals. And the bonding molecular orbitals that we will get will be an in-phase combination -- -- of our LCs, our linear combinations of atomic orbitals, with our boron atomic orbitals. That will describe the chemical bonding in our system. And we will see that it contrasts in a very interesting way with the hybridization scheme developed last time. And the key phrase to underline, here, is in-phase. And what that in-phase means is that when two positive lobes of two orbitals centered on two different atoms are juxtaposed and neighbor one another and can have good overlap of their atomic orbital wave functions, that leads to in-phase constructive interference and stabilization of the electrons associated with that newly formed bonding molecular orbital. And that stabilization is what we call the chemical bond. The analogy to that in valence bond theory is the idea that a pair of electrons associated with two nuclei in a sigma bond is more stable because it experiences, simultaneously, two positive charges. And here we are generalizing that and allowing electrons to flow over the molecule as a whole. But now we have a new concept, and that is antibonding. Antibonding molecular orbitals will be out-of-phase combinations that are repulsive and lead to high energy interactions. And when, as in the case of the problem we are considering here, the interactions occur in pair-wise sets, we will find that we get very nicely, for every bonding molecular orbital, a corresponding anti-bonding molecular orbital. Also, one of the interesting things is that if you start putting electrons into anti-bonding orbitals, if your system just happens to be so constructed as to have many electrons, such that you fill up not only the bonding molecular orbitals of electrons to make the chemical bonds, but you continue on and you have enough electrons to keep going and put them into anti-bonding orbitals, those antibonds start to cancel the bonds. And so, you will see a very nice progression of this as we study the MO theory of the homonuclear diatomic molecules, starting on Friday. And then, here is another concept that arises from the MO analysis of a molecule, and that is that certain orbitals can be non-bonding. And an example of this would be a lone pair of electrons. And it happens when an orbital or a linear combination of orbitals finds no counterpart -- -- of like nodal symmetry. These nodal properties of orbitals are very important, and I will show you later how the nodal properties are related to the energies of the orbitals, as we consider them. Having given you this preview of how orbitals are going to be able to combine in the MO theory, let's see how it actually takes place in the case of BH three. Now we are drawing another example of an energy-level diagram, where the energy is low at the bottom and rises as it goes up. And these energy-level diagrams that we are now developing for molecules are analogous to those that you studied earlier in the semester for atoms. And we are just generalizing this notion to the atoms. And what I am drawing over here is our boron 2s orbital and here is our boron 2px, 2py, 2pz orbital. Those horizontal bars just represent the energy of these orbitals in the molecule. Here I am just redrawing the boron atom. And then, over here on the right, we are going to see that we have our linear combinations that we developed. These are our three H 1s linear combinations. We found that the three hydrogens in BH three were symmetry equivalent, so we generated linear combinations. The pictures of them are over there. I can give them names. Why don't I call them D, E, and F. We have D constructed to match the nodal properties of the boron's 2s orbital. E and F were respectively constructed to match the nodal properties of the boron's 2s, 2px, and 2py orbitals. And I am showing you their relative energies. And now we need to do this issue referenced here as point three. We need to combine these things. I have these on the one hand and these on the other. Our seven atomic orbitals have been changed into four atomic orbitals and three linear combinations, so I still have seven orbitals total. And now I am going to combine these with these according to their nodal properties to generate seven molecular orbitals. And let's do it this way. We are going to take linear combination D that has been constructed to match the boron 2s orbital in terms of nodal symmetry properties, and we are going to make a bonding combination. I am going to draw these pictorially in a moment. And, in this case, those are the only two orbitals in my seven orbital system here that have that set of nodal symmetry properties. And for every bonding MO, I must have an antibonding MO. This is one of our molecular orbitals. And there is going to be a corresponding molecular orbital up here, high in energy. And this will be an antibonding molecular orbital that will be the out-of-phase combination of the boron 2s with this linear combination that I labeled D. Antibonding molecular orbitals are usually denoted with a star. We have a bonding combination and an anti-bonding combination. Now, we can form two more bonds that will spread out over the molecule because, if you recall, we had our px and py pair that served as the nodal template for our construction of linear combinations E and F. We are going to be able to match up those to form two more bonding molecular orbitals. And these will be found to be higher in energy than the first one that we formed from D. Here is a pair of bonding molecular orbitals that derive from linear combinations E and F combining in-phase with boron's px and py atomic orbitals. And there will be a corresponding antibonding combination, where we allow those orbitals to interact in an out-of-phase manner. And you will see what that means shortly. Let me put that up there and add a star to indicate that this high energy pair of molecular orbitals is an antibonding pair of orbitals. I have six orbitals now in my molecular orbital energy-level diagram for BH three. And that means I am not done because I have to have seven, and I started out with seven atomic orbitals. Look over here. 2pz was an atomic orbital on boron that did not find any way of serving as a template for making a linear combination involving the three hydrogens. And so, it comes over here as nonbonding. It has no counterpart of like nodal symmetry because of the location of those three hydrogens in the x,y-plane, which is a nodal plane for the boron pz orbital. And so, this one is nonbonding. These three orbitals up here are anti-bonding. And the ones down at the bottom, which are the lowest in energy, corresponding to being able to most tightly hold onto electrons in them are bonding molecular orbitals. And so our electrons can fill into this MO energy-level diagram in that way. We have our six electrons that come into this problem. We have boron bringing in three valance electrons and three hydrogens each bringing in one valance electron. There are six electrons to put into the diagram, filling up three of the molecular orbitals and then leaving empty pz. Let me introduce a little bit more MO language right now. This one here will be called the highest occupied molecular orbital, and this one here will be called the lowest unoccupied molecular orbital. The reason why I am drawing attention to these orbitals is that in chemistry, the chemical properties derive oftentimes from those orbitals that are in what is called the frontier orbital region. And the frontier orbitals are those close in energy to the HOMO-LUMO gap. And I will come back to this. But those highest energetically lying electrons are going to be the ones responsible for nucleophilic properties of the molecule and basic properties of the molecule and reducing properties of the molecule. Whereas, low-lying empty orbitals are going to be the ones responsible for acidic properties of the molecule or oxidizing properties of the molecule. And we will come back to that in a moment. But that is something pretty general and very useful that comes out of studying molecular orbital energy-level diagrams. Now that we have the diagram, let's see what the orbitals in the diagram look like. And I will try to do this relatively quickly. Here, let's start with the lowest lying molecular orbital in the system. This is a representation of an in-phase combination of the boron 2s plus D, where D is defined up here as that linear combination. This will be a molecular orbital having the nodal properties of a 2s orbital centered on that central atom. That is where our lowest-lying two electrons reside. Now, if you look at the LUMO over there and then go up one orbital in energy, you will be looking at this orbital, which is the boron 2s orbital minus D. And the thing that makes this out-of-phase linear combination so much higher in energy than its in-phase counterpart is the appearance, now, of a nodal surface. And this node is between the nuclei. It goes all the way around and is between the central atom s orbital and those peripheral hydrogens. And I will show you a picture of it. This is our BH three LUMO plus one molecular orbital. You can see that we have a wave function in the center of one sign. And then, as we go along any one of the B-H bond vectors from boron to hydrogen, we change phase midway along the bond from positive to negative. And that is true, no matter which of the three B-H bonds we pick to traverse along. That one has the nodal properties as drawn down there for the boron 2s interacting in an out-of-phase manner with linear combination letter D. And so, next time, I will finish up and show you what these other orbitals look like as calculated. I hope you have enjoyed this. We will see more MO theory on Friday.

Free Downloads

Video

• iTunes U (MP4 - 112MB)
• Internet Archive (MP4 - 197MB)

Audio

• iTunes U (MP3 - 11MB)

Caption

• English-US (SRT)