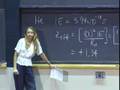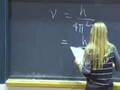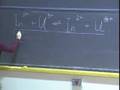Flash and JavaScript are required for this feature.
Download the video from iTunes U or the Internet Archive.
Topics covered: Ionic Bonds ‑ Classical Model and Mechanism
Instructors/speakers: Prof. Sylvia Ceyer
Lecture 12: Ionic Bonds ‑...
Related Resources
The following content is provided by MIT OpenCourseWare under a Creative Commons license. Additional information about our license and MIT OpenCourseWare in general is available at ocw.mit.edu. Last time we were talking about the general interactions that are present in a chemical bond. And we were, in particular, looking at the energy of interaction, here, as we brought two hydrogen atoms together. We were looking at that energy of interaction as a function of the distance r between these two nuclei. And we saw, of course, way out here, that the energy of interaction was minus 2,624 kilojoules per mole. As we brought the two hydrogen atoms in closer together, that interaction energy went down. It became a maximum negative value at some r. That r is the equilibrium bond length. And then, as you try to push the two nuclei closer together, the energy of interaction goes up again, so far up that when you get them really close, the energy of interaction is greater than that of the two separated hydrogen atoms. And so, in this region, the hydrogen atoms are no longer bound. Wherever this energy is lower than that of the separated hydrogen atom limit you have a bound molecule. This was the attractive part of the potential. This was the well depth or the bond association energy, measured from here to here. That is how much energy you would have to put in to pull the two hydrogens apart. This is the repulsive region of that interaction potential. We were talking about how that curve, that energy, was really the sum of three components. That energy of interaction was the sum of the nuclear-nuclear repulsion. That is, the repulsion between the nucleus of this hydrogen and the nucleus of that hydrogen. In addition to that nuclear-nuclear repulsion, there is the electron-nuclear attraction. The electron-nuclear attraction, between the electron on the original nucleus and, when the two hydrogens get close enough, the electron attraction between the other nucleus. And then, finally, there is the electron-electron repulsion. When these two hydrogen atoms come so close, the electrons now are going to repel. And so, this curve is actually the sum of those three contributions. And what we were trying to do last time is to look at the dependence of these interactions, individually, on r. And then we wanted to sum them up to see why we actually have the shape of this interaction potential that we do. We are trying to decompose this. We are trying to understand over what regions of r, which one of these interaction energies is dominant. That is what we are doing. And I think last time we started in the sense that we recognized what the r dependence was for the nuclear-nuclear repulsion. The nuclear-nuclear repulsion is just the Coulomb interaction energy between two like positive charges. That nuclear-nuclear repulsion was e squared over 4 pi epsilon nought times r. And so that component I could easily draw. And I did draw it last time, I think. This is one over r dependence. This is the e squared over 4 pi epsilon nought r. That was one of them. Now, next, these two terms are what I am going to call the electron interactions because both of them involve the electron. This one did not. This was just the nucleus. It turns out that I don't have a nice simple way to tell you what the r dependence between the nuclei will be for the electron-nuclear attraction, nor do I have a nice simple way to figure out what the r dependence will be for the electron-electron repulsion. I cannot do that without actually solving the Schrˆdinger equation. I don't have a simple way to break that down. However, what I can do is I can estimate what the sum of these electron interactions are at two extremes. Is this noisy? Can you hear me? I can see why it is noisy. I do know what it is at two extremes. I know what those sums of those interactions are for very large r, and I know what it is for r equals 0. What I am going to do is calculate it for r equals infinity and r equals 0. And I am going to then put it on this plot. And then to just estimate what the r dependence is, I am going to draw a line from that point to that point. That is the best I can do. Let's do that. Let me start with this energy of interaction at r equals infinity. I want to evaluate what the repulsive interaction is between the electrons at r equals infinity. What is that interaction energy? It is zero because the electrons are so far apart at r equals infinity that there is no interaction energy. It is the repulsive interaction. It is this kind of interaction, r equals infinity. That is going to be zero. But now this term, this electron-nuclear attraction. When the two hydrogen atoms here are very far apart, what is the energy of the electron-nuclear attraction there? I cannot hear you, so I will tell you. It is the binding energy of a 1s electron. I mean that is the energy of interaction. When r is very large, when r is infinity, the energy of interaction is just the 1s binding energy of the electron to each of its nuclei. The energy of interaction, the electron-nuclear attraction is just E sub 1s for this one. And e sub 1s for that one. In a hydrogen atom that is what it is. It is the electron-nuclear attraction. And so, right here, this is equal to 2 times E sub 1s. Now, you know that E sub 1s is equal to minus 2.18x10^-18 joules, but in kilojoules per mole, that is equal to minus 1 kilojoules per mole. And, if we have two of them, as we do, 2 times E sub 1s is minus 2 kilojoules per mole. I calculated what the electron interaction energy is at r is equal to infinity. That is way out here. This is hydrogen plus hydrogen. This is minus 2,624, the same number I got over there. Now, what we have to do -- -- is calculate what that energy of interaction is at r is equal to 0. When r is equal to 0, we have two hydrogen atoms right on top of each other. We have two hydrogen nuclei right on top of each other. That means, in our kind of thought experiment here, that the charge on the nucleus is Z equals 2. That means when the two hydrogen atoms are right on top of each other, it looks like we have a helium nucleus. And there is electron number one around it and electron number two around it. That looks like a helium atom. What is the total electron interaction in the case of a helium atom? What is the total energy of interaction there? Well, the total energy of interaction is going to be minus the first ionization energy, this is going to be the energy of interaction of the helium, for a helium atom. Because minus the first ionization energy of the helium atom is the binding energy of the first electron to the helium plus minus the second ionization energy of the helium atom. In other words, if I hid this one away, then the electron-nuclear attraction between the helium nucleus in electron two is just minus the second ionization energy of helium. Does that make sense? You are too hot to think. Okay. That is what that is. And if you look them up, the total energy of interaction is minus 7,622 kilojoules per mole. I can plot that on this graph at r equals 0. I have minus 7,622 kilojoules. Now, I have two points. I have a point over here and a point over there. I am going to draw a straight line between the two. To get my total energy of interaction, I am going to add this curve to that curve. And when I do that, we are going to get something that looks like that. The bottom line is that this shape here is determined by the competition between the electron interactions, which are always attractive. They are always negative. The electron interactions are actually the sum of an attractive term and a repulsive term, but the repulsive term is not so repulsive as to overcome the attractive term. It is always negative. Overall, the sum is still attractive. The electron interactions are attractive. This particular curve is the competition between the electron interactions, those attractive interactions and the nuclear interactions, which are repulsive. In other words, you have to get the two hydrogen atoms close enough in order for the attractive interactions to take hold. But you cannot get them so close because if you get them too close, the nuclear-nuclear repulsions set in. Where your chemical bond length is, is determined by that competition between the electron attractive interactions and those nuclear-nuclear repulsions. That is what determines the bond length. That is fundamentally, here, what determines the bond strength, the competition between these overall attractive interactions due to the electrons and the nuclear-nuclear repulsion. That was the concept, really, that I wanted to get across here, those fundamental interactions that make up this kind of curve. You are going to see this curve a lot. All chemical bonds have this kind of dependence on r, energy of interaction on r. There is one other point I want to make. That is that what we often and usually do is we reset our zero of energy. Another words, we are going to reset our zero of energy, here, so that the zero of energy corresponds to the separated atom limit. Why do we do that? Well, because this energy difference, the minus 2,624, was really the attractive interaction between the electron and its nucleus. It did not have anything to do with the attraction or repulsion between the two atoms. And so, when we want to talk only about the chemical bond and the energy changes when we make a chemical bond, it is often useful to shift our zero of energy down, so that the separated atom limit is our zero of energy. And now, everything that is negative relative to that is a bound interaction. When it gets too close, it will be a positive interaction and the atoms are no longer bound. I mean, we are not forgetting about this energy here. We know if you are calculating the total energy, it has to be there, but oftentimes we just want to talk about the relative changes of the energy of interaction as a function of r when we are concerned only with forming a bond. Make sense? Okay. That is the general phenomenon. Now, what I want to talk about is one very simple model for an ionic bond. This is a classical model. And the amazing thing about it is that this simple classical model does give us insight into the mechanism by which this bond is formed. It is a mechanism that is only going to work when you form a very ionic bond. This is particular for a very ionic bond. We want to take a look at this mechanism because it is going to give us some insight into how the bond is actually formed. We are going to look at the formation of sodium chloride, here. We have a sodium atom and a chlorine atom, and they are coming together, moving toward each other. What happens is that at a certain distance from each other, the sodium atom, believe it or not, actually ejects an electron. And that electron hooks onto the chlorine. When it does that, of course the chlorine now becomes bigger than the sodium, but now we have two charges separated. A positive and a negative ion. And there is a large attractive interaction between those two. What happens is these two ions are attracted in to each other. They are just roped right in. It is called the Harpoon Mechanism. Why does the sodium and the chlorine just pull right into each other? Well, because of that rope. That rope is that Coulomb interaction. This really happens. At some distance the sodium atom ejects that electron, and then that sodium just pulls that chlorine right into it until it gets close enough to form a chemical bond, -- and you have sodium chloride. This is a reaction mechanism that was elucidated many years ago, called the harpoon mechanicism. It is a mechanics elucidated by Dudley Herschbach, who is here at Harvard in the Chemistry Department, who has since retired. John Polanyi, who is at Toronto, and Yuan Lee, who was at Berkeley, for most of his career. They received the Nobel Prize for this discovery of this mechanism, and many other kinds of mechanism and dynamics of chemical reactions. Yuan Lee right here, this gentleman was actually my Ph.D. thesis supervisor at Berkeley. This is a simple picture, and this is exactly what is going on. This seems a little strange to you, so let's try to understand exactly how this is working. To understand this, what we are going to have to do is look at the energetics of the system. And now I am going to raise this screen here, I think. No, I don't want to. I am going to raise it a little bit. How is that? All right. What we are seeing is this gas phase sodium atom ejecting an electron to form this gas phase sodium ion, plus this electron. And of course, that is going to cost energy. The energy change is the ionization energy, which for sodium is kilojoules per mole. But, at the same time, that electron is being caught by the chlorine. And when a chlorine and an electron recombine to form the Cl minus gas phase, there is an energy release, as we saw. That energy release is minus the electron affinity of chlorine. That is equal to minus kilojoules per mole. Overall, going from a gas phase sodium atom plus a gas phase chlorine atom to a gas phase sodium ion and a gas phase chlorine ion, the overall energy change here, which is now the ionization energy minus the electron affinity, that overal energy change is 147 kilojoules per mole. Although we get some energy back when that electron attaches to the chlorine, we don't get enough energy back to compensate for having to pull the electron off of the sodium. Right now, this still looks like an overall endothermic reaction. We have to put 147 kilojoules into the system to make it go. It is beginning to seem a little peculiar. How does this work? Well, we have to remember that once we make that sodium plus and the chlorine minus, that there is that Coulomb interaction. The Coulomb interaction, bringing the sodium ion plus the chlorine ion together to make the sodium chloride, that delta E, if I take two ions, sodium and chlorine, in from infinity and bring them together at the bond length, that energy change is minus kilojoules per mole. If I add up all three reactions to get sodium gas plus chlorine gas to make sodium chloride in the gas phase, the overall energy change there is minus 445 kilojoules per mole. And, of course, the reaction is downhill. But that still doesn't give you a really good feeling for what is really going on here. To do that, let's look at an energy level diagram. You want to kill the front lights. This is going to be back and forth here. All right. What have I draw here? I have drawn the energy of interaction between a sodium atom and a chlorine atom, just like I did for hydrogen. I said all chemical bonds have this same shape of energy of interaction. Here is the bond length. 2.36 angstroms. Here is the well depth or the dissociation energy. In this case, I show it measured from here to there. It is minus delta E sub d, minus 445. Here I set the zero of energy at the separated atom limit, sodium plus chlorine. When sodium and chlorine are way out here, when r is really large, we saw that it is going to take 147 kilojoules to make a sodium ion from sodium and a chlorine ion from chlorine. That is what I calculated, right here. If the two are far apart, if you pull an electron off a sodium and put it onto chlorine, it is still going to require energy, 147 kilojoules per mole. However, I also said that when the sodium and the chlorine come close enough, the ions are pulled in close enough such that they can form a chemical bond, the energy you get back is kilojoules per mole. On this diagram, where is that? Well, that is this energy. From up here to down there is 592 kilojoules per mole. Where did I get that number, 592 kilojoules per mole? Well, I calculated it using the Coulomb potential energy of interaction, which I am calling, here, U of r sub E at this value of r. The Coulomb potential energy of interaction is right here for a point charge. If you treat the sodium as a plus one charge and you treat the chlorine as a minus one charge. All of a sudden, we are forgetting everything about the other electrons. We are just treating sodium ion and chlorine ion as two point charges. If you forget completely about the other electrons and just treat them as point charges, that is the interaction energy right here. That 592 comes from taking that expression and plugging in 2.36 angstroms. That is how much energy you get back. Now we understand the energies a little bit, but we still don't understand exactly how this electron jump process is happening. Because the way I have it drawn, here, it still looks like we have an electron jumping from sodium to chlorine way out here. And we have to put in kilojoules before we get any energy back. Well, that is not the case. And that is not the case because of this. This blue curve, here, is just the Coulomb energy of interaction. We evaluated that point, that number, from here to here using this expression. But you know that this is a minus one over r dependence. If you are way up here and you treat this as a zero of energy for a plus charge and a minus charge, one over r kind of looks like this. That is the blue curve. But you also notice that right here, you see that that Coulomb interaction is intersecting with this interaction potential between a neutral sodium atom and a neutral chlorine atom, right in there. Right at this value of r, which we are going to call r star, the potential energy of interaction from here to here is equal to the sum of this ionization energy minus the electron affinity. Right here, the electron can jump without having to put any energy into the system. You are close enough for the electron to jump because that Coulomb interaction has gotten lower, and it is right at that point when you can have that electron transfer and not have to put any energy into the system to get it to go. In other words, right here, the energy from right there to this point is minus e squared 4 pi epsilon nought r star. That energy right at that point is equal to, if I am measuring here from the top minus the quantity ionization energy of sodium minus the electron affinity of chlorine. In order to solve for this distance r at which the electron jumps, for which that electron jump is energetically allowed, I am going to set this equal to this. I know what the ionization energy and the electron affinity of sodium and chlorine are. I know everything except r star, so I am going to solve for r star. Let's do that. I am going to need the lights, here. If I rearrange that equation, r star is equal to e squared over 4 pi epsilon nought times the ionization energy of sodium minus the electron affinity of chlorine. I know what e star is. It is 1.602x10^-19 Coulomb's squared. I have a 4 pi epsilon nought. I know what epsilon nought is. And then, the difference between the ionization energy and the electron affinity I calculated over there. That is 147 kilojoules per mole or 1.47x10^5 joules per mole. But now, and this is what everybody forgets on an exam, I have to calculate r star per molecule, not per mole because per mole does not make sense. And I have this energy written here per mole, so I need an Avogadro's number up here, 6.022x10^23 per mole. Don't forget Avogadro's number when you calculate r. What is r star? Well, it comes out to be 9.45x10^-10 meters. Let's get a perspective on these distances. The sodium atom diameter is 3.8 angstroms. Chlorine atom diameter, 2 angstroms. What I am saying is that this electron can jump, or does jump from sodium to chlorine at this distance r star, which is equal to 9.45 angstroms. So, the sodium and the chlorine really are a considerable distance apart when that electron jumps. But that electron can jump because it is at that point that the Coulomb interaction is large enough here to compensate for the difference in the ionization energy and the electron affinity. And this actual number, here, was verified in these experiments by Herschbach and Lee. It actually does happen. And this very simple classical model, where we are actually treating the sodium and the chlorine as point charges, we have forgotten everything else about the electrons, that works remarkably well. Now, what this model does not give you very well is the bond energy. Because, if you look at this diagram again right in here, let me go back on here, this 147 kilojoules here, that I can look up. This 592 kilojoules, that is just the Coulomb interaction between a positive and a negative charge at 2.36 eV. That is all that is. You can calculate that. And so, therefore, if I want to calculate the bond energy of sodium chloride, the bond energy is just the difference between this energy and that energy, and that is 435 kilojoules per mole. Well, that does not come out so well in terms of the actual bond energy. The actual bond energy is kilojoules per mole. And we know why that did not come out too well. That is because this depends on all of the interactions that are much closer into the nucleus. By the time you get down here, the repulsive interactions are present. The nuclear-nuclear repulsions are present. And, in our simple model, we did not take that into account, the nuclear-nuclear repulsions. This simple model worked to get r star because r star is further out. The nuclear-nuclear repulsions have not really set in yet. Therefore, the simple model works when you are at a far distance, when r is large. But to get the bond strength, here, you are much closer in. You are at 2.36 angstroms. That is the difference in energy from here to there. We forgot about the repulsive interactions between the two nuclei, so the model is not going to work so close to the nucleus, but it does a really good job of getting r star far away from the nucleus. Again, this simple model only works for very ionic compounds, very ionic bonds like sodium chloride. It won't work very well for hydrogen chloride, for example. Questions on that? Yes? We are going to deal with entropy and Gibbs free energy changes in a week or two. Right now, what I am writing here are energy changes. I am actually dealing with single molecules. I might have, in my mind, here, energies per mole. But what I am thinking about is not an ensemble of molecules. I am actually thinking about what is happening in each individual single molecule interaction. That is why I have not talked about delta G here at all. And, in the field of chemical dynamics, that is where we want to look at individual events as opposed to a Boltzmann average of events. That is what I am talking about right here, but we are going to talk about collections of molecules and the energy changes. I am going to change my definition from delta E to delta H, the bond enthalpy, in a couple of days or so. Other questions? Okay. Well, there is one other just rather brief topic that I wanted to talk about. That is, measuring dipole moments. And then, from the dipole moments, getting out some ionic character to a chemical bond. Your book actually calls ionic bonds polar covalent bonds. And that is fine because even a very ionic bond like sodium chloride is not completely ionic, in the sense that when it is the molecule you know that it is not a point charge on sodium and a point charge on chlorine. You have an electron distribution when you are that close. And so, what you have in an ionic bond or a polar covalent bond, here, is an asymmetric charge distribution. In HCl, here, you have both electrons, on the average, being closer to the chlorine nucleus than to the hydrogen nucleus. And you have that because you have a bond between atoms with two very different electronegativities. So, that is a polar covalent bond. Now, what we are going to do is we are going to use this symbol delta here as a measure of the amount of electron transfer. Delta is the fraction of a full charge that is asymmetrically distributed. This plus delta, this delta that was on the hydrogen, is now on the chlorine. That is the interpretation of that symbol. That asymmetric charge distribution leads to a dipole moment, which is defined as q, where q is the magnitude of the charge separation, times r, where r is that charge separation. It is, strictly speaking, a vector. Q times R is what we define as a dipole moment. The units of dipole moment is Coulombs times meters. You can see that from Q times R. A dipole moment is also a vector. I have the vector on this slide going from the positively charged end to the negatively charged end. That is the way your present book does it. In your notes, I have it reversed. I have it reversed because the last time I taught this, I was using a book that used a different notation and I sent it out for Xeroxing before I noticed it was different. So, change it around. Not that it is every going to make any difference, but your book has this convention from positive to negative. This unit, though, of a Coulomb meter, is a very large unit, an inconvenient unit, so we have another unit. It is called the Debye, named after Peter Debye who first studied these polar covalent molecules. And a Debye, here, is defined as the following. It is defined as if you have a full unit charge, not a delta, moved from here to here, and the charge is separated by 0.208 angstroms, that defines this unit called the Debye. So, there are 0.208 angstroms per Debye. If you knew the fraction of charge that is separated and you knew the bond length in angstroms, and then you have our definition for a Debye, which is 0.208 angstroms per Debye, you could calculate the dipole moment in Debye. Usually what we do is not to calculate the dipole moment. We usually measure the dipole moment and calculate the partial charge distribution. Because we usually don't know this. We can measure that. We can measure the dipole moments in kind of a capacitor arrangement, where we have some molecules that have a dipole moment, a positive charge on one plate, a negative charge on the other. And, when you do that, of course, these electric dipoles are going to align. That is going to change the capacitance. If this capacitor is part of a resonant circuit, it is going to change the resonant frequent. The resonant frequency is related to the dipole moment. And, in that way, you calculate or measure the dipole moment. That is how it was originally done. However, you can now measure dipole moments very accurately by rotational spectroscopy. And we are going to look at that in a few lectures or so. But take HCl right here. Here is HCl. Here is the measured dipole moment, and here is the bond length. We can use these two pieces of information to calculate the fraction of charge distributed. And, in the case of HCl, that is about 0.18. Sometimes, we refer to this in a percentage. So, this would be 18% of a charge separation. Sometimes we say 18% ionic character in HCl. That compares to something like 70% or 80% in sodium chloride or lithium chloride. So, HCl is not anywhere near as ionic. The charge distribution is not as asymmetric as it is in sodium chloride or lithium chloride. Thank you very much for hanging in here today. It has been hot. Have a nice cool weekend. See you next Wednesday.
Free Downloads
Video
- iTunes U (MP4 - 110MB)
- Internet Archive (MP4 - 195MB)
Audio
- iTunes U (MP3 - 11MB)
Caption
- English-US (SRT)