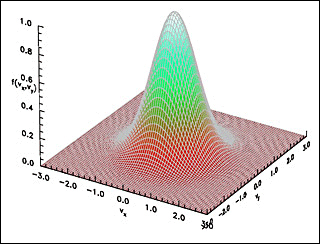
Two-dimensional Maxwellian distribution-function, typical of gas molecules or plasma particles in solutions of the Boltzmann equation and atomistic simulations. (Image by Ian Hutchinson.)
Instructor(s)
Prof. Ian Hutchinson
MIT Course Number
22.15
As Taught In
Fall 2014
Level
Graduate
Course Description
Course Features
- Captions/transcript
- Tutorial - video
- Online textbooks
- Assignments: problem sets (no solutions)
- Assignments: programming (no examples)
- Exams (no solutions)
Educator Features
Course Description
This half-semester course introduces computational methods for solving physical problems, especially in nuclear applications. The course covers ordinary and partial differential equations for particle orbit, and fluid, field, and particle conservation problems; their representation and solution by finite difference numerical approximations; iterative matrix inversion methods; stability, convergence, accuracy and statistics; and particle representations of Boltzmann's equation and methods of solution such as Monte-Carlo and particle-in-cell techniques.


