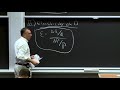Lecture 17: Making Choices Over Time

• Download English-US transcript (PDF)

[SQUEAKING][RUSTLING][CLICKING]

 

JONATHAN GRUBER: Today, what we're going to do is continue our discussion of factor markets by essentially talking about how capital markets impact real world decisions. So last time, we talked about the capital market.

We talked about, essentially, the way that firms finance their capital is by going to a pool of savings that individuals decided how much to make. Individuals make it into temporal choice on how much to save. Actually, technically, they can make a choice about how much to consume each period.

That then yields an amount of savings. And then based on that pool of savings, firms borrow at some interest rate, i, and they decide how much to invest. Now, today we're going to talk about a number of interesting applications that arise from capital markets that are important in the real world. And I'm going to start by talking about the concept of present value.

Present value. Now, the key insight when we think about capital markets is that $1 tomorrow is worth less than $1 today. $1 tomorrow is worth less than $1 today. That's because if you had the dollar today, you could productively invest it and have more than $1 tomorrow.

So $1 today is worth more, because you could do something productive with that $1 if you had it today. What that means is that dollars in different periods are worth different things. You can't just add them up. OK, the analogy I like is thinking about-- imagine you had a pound of apples, a pound of steak, and a pound of gold. You wouldn't just add them up and say, I have three pounds.

That'd be useless. You'd want to know what each is worth, and you'd want add up the dollar value of them. It's a similar thing with money received over time. You can't just say, I'm getting $1 today, $1 next year, and $1 five years from now. They aren't the same thing.

Money received at different points of time are worth different amounts, because money received in the future forgoes the possibility of investing it today. So what do we do to deal with this? Well, in economics, we deal with this by the concept of present value. Present value is the value of every period's payments in terms of today.

So the way we deal with the fact that money in different periods is worth different amounts is by what we call discounting it back to today. We essentially take future dollars and discount them back to today to get present value. We discount them, because future dollars are worth less.

So let's think of it this way. Suppose that the interest rate is 10%. Let's do an example. Imagine the interest rate, i, is 10%. And let's say that you want to have $100 next year. Next year, you want to have $100. Well, how much do you have to put in the bank today?

Well, we can solve the equation, which is that you want the amount you put in the bank today-- let's call that y-- times one plus the interest rate, because that's what you make by having it in the bank, equal to 100. That's the equation we want to solve.

The money amount you put in today, y, times one plus the interest rate. You want that to be equal to 100. So what that says is that y equals 90.9. If you put $90.90 in the bank today at a 10% interest rate, you will have $100 tomorrow.

More generally, we say that the present value of any future payment is the future value, the amount you get in the future, over one plus i to the power little t, where t is the periods in the future. So money received t periods in the future today is worth the amount you get in the future over one plus i to the t.

So that is our general formula for how we think about present value. Take all the future payments, and you discount them back to today by dividing by one plus i to the t. Now, that works well if there's one future payment coming. But what if, as in many cases, there's a whole stream of future payments coming?

Well, the logic then is the same. You just want to take each future payment and discount it by how far it is in the future. So suppose that you say that you want me to loan you $30 and that you'll pay me back $10 each of the next three years. And suppose the interest rate's 10%.

Well, I will say no to that. Because I will say, if you're going to pay me back $10 each of the next three years, then what is the present value of that? The present value is next year's $10 over 1.1, because the interest rate is 10%, plus the $10 the year after that over 1.1 squared, plus the $10 the year after that over 1.1 cubed, which if I add it up, is 24.87.

So I am losing money. If I give you $30 today and if you give me $10 back each of the next three years, I'm losing money. Why? Because if I had simply taken that money and invested it in the bank, I would've had a lot more than the $30 I'm going to get from you after three years. OK, so the money that comes in the future must be discounted back to today.

That's the key insight of present value. You can't just add it up. You've got to put it in today's terms by discounting it by the interest rate and how far into the future. Yeah.

AUDIENCE: So aside from just the change in value that's caused by interest, how do you take into consideration the fact that the value of the currency itself hasn't changed over time?

JONATHAN GRUBER: We're assuming now inflation is zero. So we're assuming right now, we're not dealing with inflation. We're assuming a world where prices don't change. We'll come back to inflation in a few minutes. But for now, just assume that prices don't change.

OK, so basically, essentially, the formula then for the present value, your general formula for present value you need to know is that present value equals-- if you get a flat stream of payment f for a number of periods, it's f times one over one plus i to the one plus one over one plus i squared plus one over one plus i cubed plus dot dot dot dot dot dot for how many periods you get it.

So if you're getting a payment f over a certain number of periods, you've got to discount it by how many periods you're going to get it. Or more generally, a common formula we'll ask you to use in this class is to think about the formula for perpetuity. A perpetuity is a flat payment you get forever.

So if we take the infinite sum of this equation, we can summarize it as present value approximately equals f over the interest rate. So if I promise you to pay you forever a certain amount f, that is worth f over the interest rate. So in other words, if the interest rate is 10%, a promise to pay you $10 forever is worth about $100.

That's just the Infinite sum. If you've read A Beautiful Mind, John Nash can do that in his head. But I can't, but that's just the formula. So basically, that is the general formula for perpetuity. And that makes life easy. We'll give you a lot of examples. Because if I say I want you to pay me for eight years in the future, I've got to write out eight terms here. It's a pain in the ass.

So what we'll say is money forever, and then you'll just use the shortcut, which is the present value for perpetuity. It's just f over i. Questions about that? Yeah?

AUDIENCE: Does this also assume the rate of change over time?

JONATHAN GRUBER: That's a great point. Yes, I'm assuming constant interest right over time. Now in reality, you'd want to have each period's interest rate here, and then you can't use this formula, because the interest rate itself is changing. Very good point.

OK, now the other way to think about this is instead of thinking just-- this will be easy for most of you, but just it's useful to think it through. Let's flip it on its head and not think about present value. Let's think about future value. This is a useful tool as well. Let's flip it on its head.

OK, so let's think about the value of a future stream of payments. Well, by the same logic we wrote before, future value-- by the same logic we wrote before, if you're going to have money that's tiers in the future, the future value of that money that you're going to get-- if you're going to basically take your money and invest it, you're going to have money that you are going to invest today and put it in the bank at some interest rate, then the future value is the amount you put in times one plus the interest rate to the t.

So I've simply reversed that formula, present value, future value. So basically, future value, if you put money in the bank at some interest rate, you're going to get to have over time that money times one plus i to the t. Now, the reason we write this formula out and flip it for you is because I want to highlight the key feature here that I want to drill into your heads.

One of the, I don't know, I guess 10 things I most care you leave this course thinking about if you're not going to major in economics or remembering-- is the beauty of compounding, which is that with a formula like this, you earn interest on your interest. If you leave money in the bank, you don't just earn interest on the initial amount you put in. You earn interest on the interest you earn over time.

OK, and this can be quite large. So let's do a simple example just to show you how big this can be and to get you all thinking about what you should do with your money when you get a job. OK, imagine you plan to work full time from age 22 to age 70. A little daunting to think about now.

Probably, most people will retire after 70 by the time you guys retire, but let's just think about 22 to 70. And let's say that you can save at a constant 7% interest rate. Inflation is zero. The interest rate is constant. Make life easy. 7% interest rate.

And let's consider two different plans you have for savings. Plan one. Plan one is that you're going to save $3,000 a year for the first 15 years that you work and then stop saving. 3,000 a year for 15 years, then you're going to leave that in the bank, leave it alone, never save anymore. Just let that money sit in the bank.

Well in that case, what will you have? Well, after the first 15 years of putting $3,000 in the bank every year, if you work out the math, you will have $75,000. $75,387 after 15 years. OK, now that's not just 15 years times-- that's bigger than 15 times 3,000, because you're earning interest along the way.

OK, that's those 15 years. Then, you're just going to let that sit there. And that's going to sit there. Remember, after 15 years-- you started working at 22. You're only 37 years old. That's going to then sit there for the next 33 years. You're not going to touch it. You're not going to save anymore.

What that means is after 33 years, this turns into $75,387 times 1.07 to the 33rd. OK, after 33 years, or $703,000. 703,010. OK, so you save $3,000. It's not a lot of money. You guys can make a lot of money.

$3,000 for 15 years, and then you never have to save again. Contrast that with a different approach. Let's say you say, look. That's stupid. I'm young. I'm going to party. I'll worry about retirement when retirement is closer. I'm going to save nothing the first 15 years, and then I'm going to save $3,000 every year.

The first 15 years I'm going to save nothing, then I'll save $3,000 every year. OK, well if you do that and do the math, you end up with when you retire, $356,800. Think about this for one second. In this case, you saved for more than twice as many years. You saved for 33 years, and you ended up with half as much.

That's the miracle of compounding. The earlier you save, the more money you can make along the way. And that's why you guys need to start saving right away. Yeah?

AUDIENCE: What was plan two?

JONATHAN GRUBER: Plan two was I do nothing the first 15 years, then I save $3,000 a year for the remaining 30 years of my career. So literally, in plan one, I save for 15 years, and then I stop. Here, I save for 33 years. But by starting earlier and using the miracle of compounding, I end up with twice as much money.

OK, now this is actually pretty-- this is one of the few things in this class my kids could understand when they were little. Because have any of you been to the Boston Science Museum? Have any of you guys been? OK, there's a little kid area, where they've got this ramp. And you can essentially drop balls down a ramp.

And one ramp is flat and then steep, and one ramp is steep and then flat. And of course, the one that's steep and then flat wins. It's faster. And that's just because of compounding. That's because acceleration is compounding.

OK, so basically, the point is that the earlier you start saving, the more money you'll have. And that's why you guys should pay attention when you're offered a job and offered a 401k and not think, retirement, I'll never retire. You say, no, I want to save now, because the more I save now, the more that will compound by the time I retire.

OK, now you can actually see this. It's not just MIT students who have to think about this but professional athletes. So probably, none of you have heard of Bobby Bonilla Any of you guys heard of Bobby Bonilla? OK, well if you were 25 years ago in this class, if you were into sports, you would have heard of Bobby Bonilla.

He was a pretty good player in his time. And he retired. But by the end of his career, he was kind of a slacker. He wasn't really worth much. He was playing for the Mets. And the Mets said, look. In 1999, they said, look. We basically want to pay you off to leave the team.

You have a contract. We're just going to basically pay the remaining 5.9 million on your contract in 1999, give you the money. Most athletes would've said great, I'm off to Vegas. Bobby Bonilla didn't. He said, well, look. I've got enough money right now, but I might need the money later.

So instead, why don't you defer the money at an 8% interest rate and pay me starting in 2011 when I'm getting close to retirement and need the money? They were like, great. That's really great. We don't have to pay you now. That's great.

Well Bobby Bonilla, by the time his payments started in 2011, they'd grown to $30 million. And every year on a certain day-- it just passed recently. They call it Bobby Bonilla. Bobby Bonilla, who's now like 70 years old, gets a million dollar check from the Mets every year. Because he was patient enough to put this off and get the virtue of compounding.

So this is sort of-- if Bobby Bonilla, a stupid baseball player, can do it, you guys can do it. So make sure that you guys are saving when you start your jobs. OK, questions about that? OK, now let's get a little bit realistic, and let's recognize that in life, prices aren't constant, but rather we have inflation.

And how does that affect our thinking here? How does inflation affect the way we think about this problem? Well, it turns out, it actually adds one step, but it's actually a pretty easy step to put in. It actually turns out that you can add inflation without doing a whole lot of work.

So let's talk about first what inflation is. Inflation is the rise in the price level year over year. Technically, the inflation rate is a percentage concept. It's the percent rise in the price level year after year. You might say, the price level of what? Of a banana? Of a computer? Of what?

Well, what the government does is they form something called the consumer price index, the CPI. Did I talk about the CPI yet? OK, I'm sorry. I just hate repeating myself. OK, so they form something called the consumer price index. What's the consumer price index?

Literally, the government every quarter, I believe-- it may be every month-- goes out and gets the prices of a basket of hundreds of goods. They literally say, what does a banana cost this month compared to last month? What does a laptop cost this month compared to last month, et cetera?

So they go out, and they price this bundle of goods. And then literally, they just ask, how has the price-- they then take a weighted average of that bundle, where the weights are consumer spending. So consumers spend a lot more of their income on housing than bananas. So the price of housing gets a lot more weight in the CPI than does the price of bananas.

Essentially, a weighted average of prices in society, and then they create an index. 1982 is normalized to one, and they just say how much in percentage terms did that weighted average bundle go up in price? OK, and you can see that in Figure 17.1.

Here's historical CPI. So basically, what you see is, this is the level of the CPI, which is sort of meaningless. What we care about is inflation, which is the year to year percentage change in the CPI. And what you can see is, basically it's going up. Prices are going up.

It went up very steeply if you look from 1970 to 1980. The slope there was much higher than the slope before or after. We had very rapid inflation in the 1970s. It then has then since flattened, and inflation has been much slower. And inflation averages about 3% a year.

OK, so basically, that's how we measure inflation. Now, the question is, how does that affect our thinking about present value if there's actually inflation? And the bottom line is, we don't care about dollars. We care about how many goods we can buy.

Therefore, it doesn't matter what's happening to how much money we have. It matters what's happening to how many goods we can buy. Therefore, what we care about is not what we call the nominal interest rate. We care about what we call the real interest rate, r, which we define as the nominal interest rate, which is what we've been talking about, we see advertised on a bank, minus the rate of inflation, which for some reason we use pi, even though that's also profits. So sorry about that.

OK, so we define the real interest rate as the nominal interest rate minus the inflation rate. The real interest rate is the nominal rate minus the inflation rate. And what the real interest rate measures is how much more I have in terms of goods I can consume, not how much more I have in terms of dollars, which actually in the end doesn't matter.

OK, so suppose that I'm going to save $100 at a 10% interest rate. Let's go a simple example. I save $100 at a 10% interest rate. OK, then next year, I have $110. But that's irrelevant. What I want to know is, how many goods can I buy next year?

So for example, let's say you spend all your money on Skittles. That's all you buy. OK, and let's say Skittles cost $1 this year. And there's no inflation, so they cost $1 next year. Then, what that means with a 10% interest rate is you can buy 10 more bags of Skittles next year.

This year, your $100 could buy you 100 bags of Skittles. Next year, your $110 can buy 110 bags of Skittles, so you are 10% richer. But now, let's say the price of Skittles goes up 10%. Well, what that means is you can only buy the same amount of Skittles next year as you could buy this year.

You could buy 100 bags this year and 100 bags next year. So it doesn't matter that you have $110 next year. Who cares? You only get the same amount of Skittles. What you care about is the goods you can buy. We wrote down utility. We didn't put dollars of utility function. We put consumption.

So the interest rate you care about is the real interest rate. If the nominal interest rate is 10% but inflation is 10%, then the real interest rate is zero. You can't buy any more goods next year. All the money you made by putting it in the bank got eaten up by how much more expensive things are. So what that means is, all the math we've done and everything we'll talk about all goes through.

You just need to be thinking about using the real interest rate, not the nominal interest rate. But otherwise, everything we've done goes through. You just need to essentially think about this in terms of how many goods you can buy, not how much money you have. Now, this isn't quite-- let me just take two minutes and do a little macroeconomics-- this isn't quite as simple as it sounds, because of course, you see in the bank, i, in the bank window, i.

You don't see pi. You don't see inflation. That's what we revealed ex post. So really, technically-- you don't know this. It's just for those who care. You don't have to know this for the test. Technically, what you really want is expected inflation. When you think of putting in the money in the bank, you know you'll learn 3%.

The real interest rate is that minus what you think inflation is going to be. So it actually becomes complicated. It's not as simple. Ex post, it's easy to find the real interest rate. Ex ante, it's not so easy, because it depends on what you think inflation is going to be. So there's some tricks there.

There's also a bunch of tricks in measuring the inflation rate. So for example, like I said, the Bureau of Labor Statistics goes out and has a bundle of 600 goods and gets their prices. But what is a good? I mean, a banana is a banana. But a laptop, what the hell is a laptop?

How much ram does it have? What's the graphics card? How fancy is the display? Well, the Bureau of Labor Statistics doesn't go out and price literally hundreds of laptops. It prices one or two. And the problem with that is the following.

Let's say you find that today, a laptop is 1,000, and tomorrow it's 1500. But it can do-- let's say today, a laptop is 1,000, and tomorrow, it's 1500. But it can do a ton more stuff. Well, we would say inflation's 50%, but that's not really right. Because the good you're consuming is not the laptop, it's the computing ability of the laptop. And that's gone up.

So to say inflation's 50% is wrong. Inflation's 50% minus the quality improvement of the better laptop you got. Think of it another way. Imagine laptop prices didn't go up, but ram doubled. Would you say you're no better off buying a laptop with twice as much ram at the same price? No, you're better off.

But our inflation concept would say, no, you're the same off. So the trick here is, it's simple in practice, and we'll pretend it's simple just to say r is i minus pi. But two tricks-- a, it's expected pi, which is hard to guess. And b, inflation is really hard to measure, because there's things like quality bias and other things. There's a whole field of macroeconomics worrying about inflation measurement, so we won't spend a lot of time on it.

But it's just sort of interesting just to talk about how at high level we go through things here. Like everything else in this class, largely, we get it right. Largely, expected inflation is not too badly modeled by last year's inflation. And quality bias, we can model and stuff. So this isn't a bad model, but it just points to some of the subtleties you have to deal with in reality when you try to implement these basic sort of formulations.

Now, with that in mind, I'm going to now say, let's assume inflation is zero again. So we'll go back and use i, and we'll use i interchangeably with r for the rest of this course unless asked differently. Unless told definitely, assume inflation is zero, so i and r are interchangeable. With that in mind, let's go to the next topic, which is taking these tools, how do we model choices over time?

How do people model? How do people make decisions over time? And there's a simple answer. So this is tricky, because if I said to you, hey, I'm going to give-- do you want $30 or $50? You would say, I want $50. But actually, you shouldn't say that. You should say, over what period of time am I getting the 30, and over what period of time am I getting the 50?

If it's today, I want 50. But if the 50 is 20 years in the future and the 30's today, I might want the 30. What that means is, you have to evaluate choices in present value terms. You can't just add up the money, you have to evaluate those choices in present value terms. And then, you need to pick the option with the highest present value.

So once again, let's come back to athletes, because athletic contracts deal with this all the time. Let's imagine an athlete considering two different contracts. Contract one, contract a, pays $1 million today. Contract b pays 500,000 today and 1.5 million in 10 years.

OK, now when you read it in the newspaper, you'll see this guy got offered a million, this guy got offered two million. That's what the newspaper will say. But that's wrong, because these are paid at different periods of times, so they're different amounts. Indeed, the present value of the first contract is what?

$1 million. It's today. What's the present value of the second contract? Someone tell me how I'd write that down. I'd write down the present value of the second contract. Yeah?

AUDIENCE: 500,000 plus 1.5 million over one plus whatever the interest is based on the time.

JONATHAN GRUBER: Exactly. Which is one plus the interest rate to the 10, because you're getting it in 10 years. So essentially, that means that whether it's a better deal or not depends on the interest rate. Indeed, if the interest rate is 7%, and the interest rate is 7%, this has a present value of 1.3 million. So it's a good deal.

If the interest rate was 14%, then this deal would have a present value of 0.9 million. So it's a worse deal. So whether or not this a better or worse deal depends on the interest rate. Why is that?

Why is this a worse deal the higher the interest rate?

AUDIENCE: Because if he'd gotten the money earlier, he wouldn't have benefited from being able to collect that interest earlier.

JONATHAN GRUBER: Exactly, he could have invested it earlier, gotten the compounding, and had way more money in 10 years. So the higher the interest rate, the more you want to get your money upfront and save it, the less valuable is money in the future. Now, essentially, what this says is that you have to always use present value to bring things into current dollars.

Now, this is not an abstract concept. Let's take Max Scherzer, who's a pitcher with the Washington Nationals. Max Scherzer a couple of years ago signed a seven year $210 million contract, which he was able to brag was the second highest ever signed by a pitcher and the 10th highest contract ever signed by any baseball player. Seven years, 210.

But in fact, that contract was not-- we're going to pay you $30 million a year for seven years. It was, we're going to pay you $15 million a year for 14 years. You're going to play for only seven. We're going to pay you over 14 years. So in fact, in present value terms, that was worth somewhat less.

If you use the current interest rate when he signed it which was about 4.7%, it was only worth actually 166 million. Not too shabby, but suddenly it drops to the 20th most valuable baseball contract and about fourth among pitchers. So Max Scherzer was able to feel better about himself that he signed this valuable contract, but in fact, it was worth less than he thought.

I mean, shed no tears for Max Scherzer. He's doing fine. But it was worth less than he thought. Or maybe an example that's more in our mind with the Mega Millions-- think about a lottery winner. So if a Mega Millions winner gets $290 million, which sounds like a great deal, that's paid out over 20 years. So it's not $290 million, it's 14 and and a half million for each of 20 years.

So in present value terms, if we think about a 7% interest rate, then that's not 290 million, it's 164 million. Once again, not too shabby. OK, but a lot less than the advertised amount. So we have to take these dollars and put them in present value.

So now, here's an interesting question for that. If you look at the recent lottery, you had a choice of one person won 1.6 billion, one person one that, a person in South Carolina won that. And they were given a choice of the 1.6 billion, which is paid actually over 30 years, or a lump sum they could get right away. How should they decide which of those options to take?

How should they decide whether to take the 1.6 billion paid over 30 years, so 1.6 over 30, paid in equal installments over time versus just getting a lump sum today. Not of 1.6 million, but a lower amount. How should they think about that?

AUDIENCE: They should evaluate the present value.

JONATHAN GRUBER: They should add the present value. They should say, well, what do I think the interest rate is going to be? If I think it's going to be really low-- let's say the interest rate is going to be zero. Then which deal should I take?

AUDIENCE: [INAUDIBLE]

JONATHAN GRUBER: I should spread it out over time, because the money in the future is worth the same as today, so I might as well take the 1.6 billion. But if I think the interest rate is going to be higher, than I should take the money upfront and invest that money and earn my own interest. So essentially, it becomes a debate of what you think the interest rate is versus what the state thinks the interest rate is.

They're setting those two to be equal under some assumption of the interest rate. I don't know what number they chose. Whatever number they chose. It was some number they chose, because this is our assumption of the interest rate. You've got to decide, do you think the interest rate's going to be higher or lower?

If you think it's going to be higher than the state thinks it is, then you want the money upfront, and you'll invest it. If you think it's going to be lower, than you should take the money over time, because the state is giving you basically a better deal. Do you understand that?

OK, so now armed with this, let's go and think about how do firms make investment decisions? How do firms make investment decisions? Remember, investment is about the delay of current consumption for future assumptions. It's about putting some aside today by spending money on a machine which will deliver you benefits in the future.

Now, this adds one wrinkle to what we've done so far, which is that we've only talked about money that's always positive, some amount you get get in the future. When you're making an investment decision, it's a little bit more complicated, because you're actually spending money today to make money tomorrow. So in that case, we talk about we call net present value, which is the same thing, it just allows for negative values.

Net present value, which is essentially saying in every period, you want to account for the cost of that period and the benefits of that period, and you want to invest only if the net present value is greater than zero. So for example, think about a project that has a stream of payments in every period of r sub i, every period it's got a stream of payments of r sub i, and a set of costs that's c sub i.

The costs are the upfront investments, maybe the maintenance of the machine. Whatever it costs to run it. Then, the net present value of that investment is r0 minus c0, comma r1 minus c1 over one plus i-- over one plus i plus r2 minus c2 over one plus i squared plus dot dot dot for as many periods as the investment lasts.

That's the net present value of an investment. So basically, what you want to ask is, take each period's costs of benefits into account. On net, is it greater than zero? OK, so the key point is that basically, sometimes investments which have upfront costs can be valuable as long as the long run benefits are large enough.

So if you think about an investment that's got 100-- so think about a simple, trivial example of the first period you buy a machine, and it costs $100. So c0 is 100. And let's say for every period thereafter, that machine will deliver you revenues, revenue i-- revenues for i greater than zero-- of 200.

But it will have maintenance costs, costs i greater than zero, of 50. So what's the net present value? Well, the net present value is simply minus 100. And let's say the machine is going to last forever. Minus 100 plus 150 over i.

Think about that for a second. Basically, what we're saying is, you're throwing 100 at it today, so that's negative. But every period in the future, you're going to net $150, because you're going to make $200 and you have $50 maintenance costs. And we have the formula, so we just apply the formula for perpetuity.

We have a set of future payments of $150, so your net present value is minus 100 plus 150 over i. So let's think about, look at this formula for a second. What does that say? What is the relationship between whether the firm's going to want to invest and the interest rate?

What does this imply the relationship is between a firm's desire for investment and the interest rate? If the interest rate goes up, will firms want to do more investment, or less investment, and why? Will firms want to be more eager to buy machines or less eager to buy machines, and why, as the interest rate goes up? Yeah.

AUDIENCE: They'll be less eager.

JONATHAN GRUBER: Less eager.

AUDIENCE: [INAUDIBLE]

JONATHAN GRUBER: They'll be less eager. Say it again, because why?

AUDIENCE: Because if they're borrowing.

JONATHAN GRUBER: Yeah, or I think an easier way to think of borrowing, just think about, they've got a bunch of money. Apple's got a bunch of money today they're sitting on. If they buy the machine, they're going to get this return.

If they don't buy the machine, they could put it in the bank or invest it in Apple stock and get some interest rate, i. The higher the interest rate, the less they want invest. Or think of it this way. The interest rate is the opportunity cost of investment.

The more the firm invests in their machines, the less they can earn saving through some other mechanism. And the price that they pay by foregoing that other savings is i. Yeah.

AUDIENCE: So does that mean if you're looking at a machine, it's better to try to then push the cost further into the future, because then they can't also divide by the [INAUDIBLE] sign?

JONATHAN GRUBER: Basically, you always want to try to make as much money early as you can and make the cost as late as you can. For any given amount, you'd absolutely like to do that. That's why we'll talk about this in my public finance class. We talk about why, when you're paying taxes, you always want to try to find shenanigans that allow you to pay your taxes later.

So for any given amount of taxes, the later you pay it, the less it costs you, because you get to earn the interest along the way and then pay it later on. This is a key macroeconomic concept. You'll often hear in the news, high interest rates are bad for business.

And you might have thought, why is that? This is why. High interest rates are bad for the economy, you'll hear. Why are high interest rates bad for the economy? You might say, wait a second. That makes no sense. A high interest rate means I earn more on my savings. Why is that bad for the economy?

It's bad for the economy, because it lowers the demand for capital. Because the higher the interest rate, the less firms actually want to invest. The more they just want to stock their money away in the bank. So that is the key question.

Now, then let me ask another question. If you're a firm, what's the right i to use? If you're a firm thinking about this investment decision, we know the higher i is, the less you want invest. But what is i? Once again, forget inflation. Inflation is zero.

What's the right what we call firm discount rate? The discount rate is the amount by which firms are going to discount future dollars to bring them back to today. How does the firm think about what i to use? What i should the firm use?

Your Apple. Yeah, go ahead.

AUDIENCE: [INAUDIBLE] i that makes that positive, right?

JONATHAN GRUBER: Well, they don't want to invest unless it's positive. That's a good point. But the way that is, they write down the math, and they plug in an i. What i do they plug in? Yeah.

AUDIENCE: Is it published by the government?

JONATHAN GRUBER: Well, i might be. But there's not one answer. What's the general answer the firm wants to use? What's the general answer? That the firm-- if you're a firm thinking about making an investment, you want to discount that investment. What do you want to discount it by? Yeah.

AUDIENCE: The opportunity cost of the next best return.

JONATHAN GRUBER: The next best thing you could do. So if I'm thinking about buying this machine, when I discount it, I want to think about what's the next best thing I could do with that money? That's the discount rate I want to use. So in a world where firms either can buy a machine or put it in the bank, it's easy. It's the bank interest rate.

But life's not that easy. Firms have dozens of investments. So for every investment, you want to discount it by the next best thing you can do with the money. It's the concept of opportunity cost. That's why it's the very first thing we taught in this class.

Opportunity cost is always what drives things. Questions about that? Now, this isn't just for firms. This same math applies to consumers as well. Let's think about me.

A number of years ago, I had to decide whether to insulate my ancient house. Let's write down the numbers to think how this worked. I had heating bills at that time, back when gas was cheaper, of about $2,000 a year was my heating bills for the house. The best estimate I could get was that if I insulated my house, I would lower my heating costs by 25%.

So my heating costs would fall by $500 per year if I escalated my house. But to insulate my house, I had to pay the guy to insulate it. And the insulation cost $4,000. How do I think about whether I should insulate or not? How do I think about that decision?

What equation should I write down? Yeah.

AUDIENCE: Minus 4,000 plus 500 over i.

JONATHAN GRUBER: Exactly. I should say, I'll assume I'm going to own the house forever, or at least long enough that I can treat it as forever. And I write down that formula. And what that formula says is that if I think the interest rate is less than 12.5%, I should insulate. If I think the interest rate is more than 12.5%, I should just invest the money and use the returns that I invested to pay my higher heating bills.

So it all depends on what the interest rate is. So that's why-- I did it. I insulated. So the same logic we can think of is basically, essentially the same idea as firms. You want to think about the upfront costs and the long run returns. And here's a fun economics question.

What if I don't intend to hold the house forever? I would argue I should still use this formula. Why? Yeah?

AUDIENCE: Because whenever you decide to sell the house, you increase the value.

JONATHAN GRUBER: Exactly, because I'm increasing the value of an asset that I'll then sell. So presumably, by insulating, I've raised the price of my house. How much have I raised it by?

Exactly 500 over i, so I'm going to insulate and sell next year. I should still insulate, because I should get 500 over i more dollars for my house. So in fact, it doesn't matter. If you can sell an asset, then actually your horizon is always infinite.

It's not just the short horizon, which is kind of an interesting insight. So the last thing I want to talk about is the fact that these decisions are not just relevant-- you guys are like retirement, business machines, insulation-- god, you're old, John. I don't care about any of this stuff.

Well, let's talk about something you care about, which is going to college. Let's talk about your decision. You've already made it, but you've got a little sibling, and they're deciding whether to go to college. And they're not going to go to MIT. They're going to go to a more typical school.

And they've got to decide whether to go to college. Well in fact, their decision is an investment decision, just like any other investment decision. What they're investing in is what we call their human capital.

When you get education, you're investing in yourself, just like you invest in a machine. Because you are, you hope, raising the value of what you can do, of what you can earn, by investing in learning stuff. Well, that human capital investment has the same features of any other investment.

There's an opportunity cost, which is what? What's the opportunity cost of investing your time in going to college-- what's the opportunity cost of going to college?

AUDIENCE: You could get a job.

JONATHAN GRUBER: You could-- there's two. One is, you can get a job.

AUDIENCE: You could also invest your tuition.

JONATHAN GRUBER: You could not pay tuition. So if you think about going to college, you're sacrificing two things. All that money you're paying, you could basically invest instead of giving it to some college. And you could be out earning money instead of sitting here listening to me.

OK, so if you think about that, it actually becomes a harder decision than you might think. So let's think about a simple example. Let's imagine that if you don't go to college, you work from age 18 to 70. And if you do go to college, you work from age 22 to 70.

So we're going to ignore grad school. Four years of college, you either start working at 18, or you start working at 22. And let's say college costs $35,000 a year. Obviously, not MIT. OK, let's say college costs $35,000 a year. And let's say that if you worked starting in high school, you could have earned $20,000.

 

You could have started at $20,000 if you'd gone to work at age 18. Well, we can actually graph what this looks like in figure 17.2 17.2. If you think about age 18, from age 18 to age 22, that's the green area. If you go to college, you give up the $35,000 in tuition and the 20,000 you could have earned.

The bottom line is basically-- the red line is your lifetime earnings if you go to high school, if you don't go to college. The blue line is your lifetime earnings if you do go to college. Empirical estimates suggest that at age 22, the typical college graduate earns $45,000. Yes, you're not the typical college graduate.

The typical college graduate earns $45,000. And the typical high school non-college education person earns $28,000. So at age 22, you come out of college earning 45, and if you'd not gone to college, you earn 28. Not you, but a normal person.

But moreover, knowledge you earn more when you leave college, your earnings grows faster. So if you're college educated, it not only means you earn 17,000 more at 22, it also means your earnings grows faster. So that by age 51, the average college educated person earns $80,000, while the average high school person earns $45,000.

So what you see here is, the blue line starts above the red line and the gap widens over time. And maybe, I would have learned to clip this on if I hadn't gone to college. OK, so the gap widens over time. So how do we think about this decision?

Well, the cost is the green area. The cost is over four years, you could have earned money and you wouldn't have had to pay tuition. The benefit is the yellow area. Over that entire time after graduation, you're making more money. Now obviously, in terms of size, the yellow area is much, much bigger than the green area. But the yellow area comes later.

That's the key thing. So if I look and say, look, it's obvious-- before this lecture, you might say, well, it's obvious you should go to college. Look, the yellow is way bigger than the green. But that's not necessarily true, because the green comes now, and the yellow comes later.

Indeed, if you look at the table, this actually shows the net present value of going to college and high school. And what this shows is at low interest rates, you're much better off going to college. So if there's interest rate, then college is a much, much better deal. Your net present value of earnings if if you go to college is 2.6 million, while it's only 1.6 million if you don't go to college.

But once the interest rate gets above 8%, it suddenly becomes a worse deal to go to college. That is at only a 9% interest rate, which existed not that long ago in our history. It was actually a worse deal for the average person to go to college. Yeah.

AUDIENCE: Isn't that not accounting for financial aid, though? Because if you didn't have the 35,000 to spend, you wouldn't have been able to invest it?

JONATHAN GRUBER: Well, actually, it's interesting. It depends on the form of financial aid. Why? Someone tell me why it's dependent on the form of financial aid? Financial aid comes in different forms. So why does it depend on how you get the financial aid? Yeah.

AUDIENCE: Because if you have to pay it back.

JONATHAN GRUBER: If it's a grant, then yeah, you should just take that out of the cost. But if it's a loan, it depends what interest rate you get the loan at. If the loan is at the market interest rate, then it's no different. But that's a great point, which is why college financial aid comes in two forms.

Grants for very low income people, and low interest loans for other people. Why do we give low interest loans for college? Because of this graph. Because we're saying, we think people need to invest in their education. We're afraid that if they faced a regular interest rate, they won't be willing to do it, because the green would be bigger than the yellow.

So we're actually going to subsidize their interest rate. So you might have thought to yourself, sort of a weird way to get people to go to college is to have a lower student loan interest rate. But in fact, it makes perfect sense. By having a student loan interest rate that's lower than the market rate, you encourage people to go to college, because essentially you lower this discount rate, at least on the part that's tuition payments.

 

So that's actually very exciting way to think about and a very important part of public policy is how we set the interest rate on student loans. For any of you-- how many of you guys have a student loan? Do you know? Any of you guys have a student loan?

You might not know. Anyway, I set the interest rate on that student loan. So thank you. Actually, when I was in the government, I was in the government 14 months, the Clinton Administration. It was super fun. But looking back, I only got one thing done, which I got to set the interest rate for student loans. So that was kind of fun.

But otherwise, it was just a lot of fun being there. So anyway, let's stop there, and we will continue. What's today, Wednesday? So no class on Monday. That's Veterans Day, so we'll meet in a week, next Wednesday.