On Conic Sections, Conservation of Angular momentum and Kepler's Second Law

Consider a cone whose axis is the z-axis, and whose points obey the equation

z = r

A conic section is any intersection of this surface with a plane.

A plane is defined by one linear equation in the variables x, y and z. Without loss of generality we choose that equation so that it has no y term, and the coefficient of x is positive or 0.

It then can be written as

z = ax + b

where a is non-negative.

The intersection of such a plane with our cone is then described by the two dimensional equation

r = ax + b

There are two general cases here which lead to two kinds of curves. These correspond to the parameter a being less than or greater than 1.

There are a number of special cases that occur when the parameter a is 1 or 0 or b is 0.

We can reduce the equation here to one involving x and y along by squaring both sides. The result is

x² + y² = r² = a²x² + 2abx + b².

You will observe that when a is less than 1, this curve becomes an ellipse with center on the x-axis, but not at the origin. The origin is said to be a focus of the ellipse.

The ellipse is of course symmetric about its center. Therefore it has another focus on opposite the origin from the center.

When a is strictly greater than 1 the curve is a hyperbola and the origin is again a focus of that hyperbola.

Exercises:

1. What other curves do you get as conic sections in the various special cases?

2. How far is the origin from the center of the ellipse for an ellipse having parameters a and b?

3. For an ellipse as defined here, the x-axis and y-axis are axes of symmetry, in that if the ellipse is rotated 180^o about either axis, it does not change. Which of these is the major (longer) axis, and which the minor axis?

Newton showed that a conic section (or one sheet of a hyperbola) can be an orbit for a planet subject to the inverse square attraction of a sun.

This is by no means an obvious fact. His equations of motion describe the second derivatives of x and y with respect to t. The implications of these about the orbit are not easy to see.

One way to look at it is as follows. If you consider an ellipse you can compute as a function of position (x, y) on it.

If we can do the same for a solution to Newton's equations we can show ellipses with appropriate parameters have the same dependence and are therefore solutions.

To do so we notice that Newton's equations have two standard constants of the motion: angular momentum (whose conservation is essentially Kepler's Second Law) and energy.

With the definition that the vector p is mv with m particle mass and v velocity, then the cross product of p and the position vector r is proportional to the cross product of v and r. Its time derivative is then proportional to the cross product of the acceleration or force and position which is zero for any "central force".

This statement, called the conservation of angular momentum. In our context tells us

for some constant A.

The conservation of energy is the statement that the energy of this system is also constant.

It can be expressed as

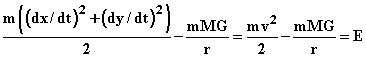
for some constant E.

These two equations involve dx, dy, dt, x and y.

You may use the first equation to express dt in terms of the others, then substitute it into the second to get a quadratic equation for in terms of x and y and A and E (remembering that r is ).

By substituting your known relation of for a conic section into this equation you can identify the values of a and b which will obey that equation for given A and E.

I personally found this or whatever other way given for showing that conic sections are solutions so long and boring when I was exposed to it, that I remember nothing of it and I refuse to carry this any further.

Exercises:

4. Find for a conic section in terms of the parameters a and b.

5. For what values of energy E is the orbit of the planet(?) bounded?

You can examine orbits for this system using a spreadsheet as indicated in Section 33.4 and verify for yourself that the claim here is really true.

Anyway, Newton not only invented calculus but he used it to show that Kepler's Laws all follow from the inverse square central force law of gravity.