22.2 Measures on Surfaces and Stokes Theorem

Suppose now we have two adjacent but not overlapping areas, say rectangles, in a plane. We ask: what entity can we define on the boundaries of each rectangle that will be additive, like the difference of F is on a curve?

What we need is that the contributions to our entity from the common boundary of the two rectangles cancel each other out.

There is an easy way to achieve this: we can integrate something around the outer bounding perimeter of the rectangle, in some standard direction.

We always choose the counterclockwise direction as the positive one.

If we do so we will integrate up the internal boundary between two adjacent rectangles in one and down that boundary in the other and it will contribute nothing, and we will have our additivity.

The next question to consider is, what kind of entity should we integrate over the boundary of our rectangle (or other region)?

We could in principle integrate either a scalar or vector field in this way; we saw in the last chapter that the line integral of a vector field was the most natural generalization of the ordinary integral to a path integral

The natural additive boundary defined entity for an area is then the integral around its boundary of the line integral of the dot product of some vector v with the path tangent vector

This entity will be additive on areas A for any vector v for which it is defined, in the plane, or for that matter on any surface that is locally planar, and even on any surface that consists of pieces that are locally planar.

The symbol that we use for an integral of this kind, with a circle on the integral sign, is meant to indicate that we are integrating all around the boundaries of the piecewise locally planar region A.

We now ask what is this integral, when A is a rectangle with axis parallel sides? (By the way we can always rotate our coordinates so that any rectangle will, after the rotation sides will be axis parallel.)

We assume we are working in three dimensional space.
Then our integral consists of two parts, the vertical and horizontal segments of the integral around A as illustrated here.

We choose our rectangle to have corners (x, y) and (x + dx, y + dy) for infinitesimal dx and dy.

Then the integral up the right side and down the left of vdl will contribute

(v_y(x + dx, y) - v_y(x, y))dy

while the integral from right to left across its top and from left to right across its bottom contributes

(v_x(x, y) - v_x(x, y + dy))dy

Observe that for differentials, we can rewrite A(q + dq) - A(q) as Applying that here and adding the two contributions above we find that our integral around our infinitesimal rectangle is

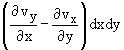
In our coordinate system here, the z direction is normal to the plane of the rectangle, and you will observe that what we have here is the component of the curl of v normal to the rectangle, multiplied by the area element of the rectangle

And now we have our Fundamental Theorem. For area integrals in the plane it is called Green's Theorem. For general sufficiently piecewise smooth surfaces it is called Stokes Theorem

This result holds for A any region on a surface that can be broken up into small rectangles, by the additivity of both sides of this equation.
Actually, you can, with slightly more labor (which we omit), derive the same infinitesimal result for triangles as well as rectangles. Thus the conclusion holds as well for any region that can be reasonably broken up into tiny non-overlapping rectangles and triangles.

You will observe that the curl of v arose naturally in this theorem. In fact, the nature of this theorem is the main reason we are interested in the curl.

This theorem has a number of immediate consequences.

First, if the curl of v is 0 on a surface, the line integral of v from one point to another in it will be independent of the path, so long as the path stays within it.

Second, if you can evaluate the line (or path) integral of v from one point to another along one path, you can deduce its integral along another path, by integrating its curl on any surface whose borders are the the two paths.