15.5 Torsion

We can go further. The next quantity of interest is how much the plane of curvature "twists". This is measured by the torsion of the curve, which is the magnitude of the derivative of the normal to the plane of curvature with respect to distance on the curve.

This derivative can be calculated straightforwardly. B(t), as already noted, can be written according to

By the chain rule we have

Notice further that we can apply the product rule to the cross product to get 

and the latter term is 0.

We also have

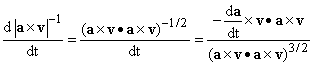
We can also use the identity

Putting all this together  we get

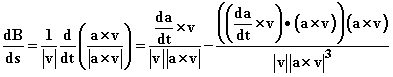
We find then that the torsion is the magnitude of this and hence of the component of and divided by the area of the parallelogram formed by a and v.

Nobody remembers this formula. Be content with being aware it exists, that you could compute it yourself if you ever were forced to do so, and that it can be set up to be calculated automatically with a spreadsheet.

Exercises:

15.4 As a test of your manipulative skill, see if you can wade through the steps above yourself and get the correct answer (which I hope is the one given above).

15.5 Set up a spreadsheet that for the curve and domain of Exercise 15.3, and compute its curvature and torsion at representative values of the parameter, as well as the coordinates of the curve at the same values. Where is the torsion the greatest in your range?